Difference between revisions of "User:Jon Awbrey/GRAPHICS"
Jon Awbrey (talk | contribs) |
Jon Awbrey (talk | contribs) (+ Miscellaneous Examples (Reduction 8:1)) |
||
| (323 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
| + | ==Animations== | ||
| + | |||
| + | ===Riffs 1 to 60=== | ||
| + | |||
| + | {| align="center" | ||
| + | | [[Image:Animation Riff 60 x 0.16.gif]] | ||
| + | |} | ||
| + | |||
| + | ===Rotes 1 to 60=== | ||
| + | |||
| + | {| align="center" | ||
| + | | [[Image:Animation Rote 60 x 0.16.gif]] | ||
| + | |} | ||
| + | |||
| + | ==Image Gallery== | ||
| + | |||
| + | ===Reduction 6:1=== | ||
| + | |||
| + | ====Cacti==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="50%" | '''Image''' | ||
| + | | width="50%" | '''Scale''' | ||
| + | |- | ||
| + | | [[Image:Rooted Node Big.jpg|20px]] || 117 px <br> ↓ <br> 20 px | ||
| + | |- | ||
| + | | [[Image:Rooted Edge Big.jpg|20px]] || 117 px <br> ↓ <br> 20 px | ||
| + | |} | ||
| + | |||
| + | ====Riffs==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="15%" | '''Integer''' | ||
| + | | width="70%" | '''Riff''' | ||
| + | | width="15%" | '''Scale''' | ||
| + | |- | ||
| + | | 1 || || | ||
| + | |- | ||
| + | | 2 || [[Image:Riff 2 Big.jpg|20px]] || 117 px <br> ↓ <br> 20 px | ||
| + | |- | ||
| + | | 3 || [[Image:Riff 3 Big.jpg|40px]] || 240 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 4 || [[Image:Riff 4 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 5 || [[Image:Riff 5 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 6 || [[Image:Riff 6 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 7 || [[Image:Riff 7 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 8 || [[Image:Riff 8 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 9 || [[Image:Riff 9 Big.jpg|40px]] || 240 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 10 || [[Image:Riff 10 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 11 || [[Image:Riff 11 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 12 || [[Image:Riff 12 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 13 || [[Image:Riff 13 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 14 || [[Image:Riff 14 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 15 || [[Image:Riff 15 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 16 || [[Image:Riff 16 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 17 || [[Image:Riff 17 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 18 || [[Image:Riff 18 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 19 || [[Image:Riff 19 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 20 || [[Image:Riff 20 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 21 || [[Image:Riff 21 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 22 || [[Image:Riff 22 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 23 || [[Image:Riff 23 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 24 || [[Image:Riff 24 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 25 || [[Image:Riff 25 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 26 || [[Image:Riff 26 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 27 || [[Image:Riff 27 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 28 || [[Image:Riff 28 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 29 || [[Image:Riff 29 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 30 || [[Image:Riff 30 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 31 || [[Image:Riff 31 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 32 || [[Image:Riff 32 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 33 || [[Image:Riff 33 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 34 || [[Image:Riff 34 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 35 || [[Image:Riff 35 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 36 || [[Image:Riff 36 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 37 || [[Image:Riff 37 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 38 || [[Image:Riff 38 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 39 || [[Image:Riff 39 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 40 || [[Image:Riff 40 Big.jpg|135px]] || 816 px <br> ↓ <br> 135 px | ||
| + | |- | ||
| + | | 41 || [[Image:Riff 41 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 42 || [[Image:Riff 42 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 43 || [[Image:Riff 43 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 44 || [[Image:Riff 44 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 45 || [[Image:Riff 45 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 46 || [[Image:Riff 46 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 47 || [[Image:Riff 47 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 48 || [[Image:Riff 48 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 49 || [[Image:Riff 49 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 50 || [[Image:Riff 50 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 51 || [[Image:Riff 51 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 52 || [[Image:Riff 52 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 53 || [[Image:Riff 53 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 54 || [[Image:Riff 54 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 55 || [[Image:Riff 55 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 56 || [[Image:Riff 56 Big.jpg|135px]] || 809 px <br> ↓ <br> 135 px | ||
| + | |- | ||
| + | | 57 || [[Image:Riff 57 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 58 || [[Image:Riff 58 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 59 || [[Image:Riff 59 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 60 || [[Image:Riff 60 Big.jpg|115px]] || 672 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 64 || [[Image:Riff 64 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 81 || [[Image:Riff 81 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 105 || [[Image:Riff 105 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 128 || [[Image:Riff 128 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 165 || [[Image:Riff 165 Big.jpg|135px]] || 816 px <br> ↓ <br> 135 px | ||
| + | |- | ||
| + | | 256 || [[Image:Riff 256 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 512 || [[Image:Riff 512 Big.jpg|65px]] || 384 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 2010 || [[Image:Riff 2010 Big.jpg|185px]] || 1104 px <br> ↓ <br> 185 px | ||
| + | |- | ||
| + | | 2500 || [[Image:Riff 2500 Big.jpg|90px]] || 528 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 65536 || [[Image:Riff 65536 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 802701 || [[Image:Riff 802701 Big.jpg|210px]] || 1248 px <br> ↓ <br> 210 px | ||
| + | |- | ||
| + | | 123456789 || [[Image:Riff 123456789 Big.jpg|220px]] || 1296 px <br> ↓ <br> 220 px | ||
| + | |} | ||
| + | |||
| + | ====Rotes==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="15%" | '''Integer''' | ||
| + | | width="70%" | '''Rote''' | ||
| + | | width="15%" | '''Scale''' | ||
| + | |- | ||
| + | | 1 || [[Image:Rote 1 Big.jpg|20px]] || 117 px <br> ↓ <br> 20 px | ||
| + | |- | ||
| + | | 2 || [[Image:Rote 2 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 3 || [[Image:Rote 3 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 4 || [[Image:Rote 4 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 5 || [[Image:Rote 5 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 6 || [[Image:Rote 6 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 7 || [[Image:Rote 7 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 8 || [[Image:Rote 8 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 9 || [[Image:Rote 9 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 10 || [[Image:Rote 10 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 11 || [[Image:Rote 11 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 12 || [[Image:Rote 12 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 13 || [[Image:Rote 13 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 14 || [[Image:Rote 14 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 15 || [[Image:Rote 15 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 16 || [[Image:Rote 16 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 17 || [[Image:Rote 17 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 18 || [[Image:Rote 18 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 19 || [[Image:Rote 19 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 20 || [[Image:Rote 20 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 21 || [[Image:Rote 21 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 22 || [[Image:Rote 22 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 23 || [[Image:Rote 23 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 24 || [[Image:Rote 24 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 25 || [[Image:Rote 25 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 26 || [[Image:Rote 26 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 27 || [[Image:Rote 27 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 28 || [[Image:Rote 28 Big.jpg|130px]] || 761 px <br> ↓ <br> 130 px | ||
| + | |- | ||
| + | | 29 || [[Image:Rote 29 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 30 || [[Image:Rote 30 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 31 || [[Image:Rote 31 Big.jpg|40px]] || 233 px <br> ↓ <br> 40 px | ||
| + | |- | ||
| + | | 32 || [[Image:Rote 32 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 33 || [[Image:Rote 33 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 34 || [[Image:Rote 34 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 35 || [[Image:Rote 35 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 36 || [[Image:Rote 36 Big.jpg|145px]] || 857 px <br> ↓ <br> 145 px | ||
| + | |- | ||
| + | | 37 || [[Image:Rote 37 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 38 || [[Image:Rote 38 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 39 || [[Image:Rote 39 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 40 || [[Image:Rote 40 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 41 || [[Image:Rote 41 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 42 || [[Image:Rote 42 Big.jpg|145px]] || 857 px <br> ↓ <br> 145 px | ||
| + | |- | ||
| + | | 43 || [[Image:Rote 43 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 44 || [[Image:Rote 44 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 45 || [[Image:Rote 45 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 46 || [[Image:Rote 46 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 47 || [[Image:Rote 47 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 48 || [[Image:Rote 48 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 49 || [[Image:Rote 49 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 50 || [[Image:Rote 50 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 51 || [[Image:Rote 51 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 52 || [[Image:Rote 52 Big.jpg|145px]] || 857 px <br> ↓ <br> 145 px | ||
| + | |- | ||
| + | | 53 || [[Image:Rote 53 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 54 || [[Image:Rote 54 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 55 || [[Image:Rote 55 Big.jpg|80px]] || 473 px <br> ↓ <br> 80 px | ||
| + | |- | ||
| + | | 56 || [[Image:Rote 56 Big.jpg|130px]] || 761 px <br> ↓ <br> 130 px | ||
| + | |- | ||
| + | | 57 || [[Image:Rote 57 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 58 || [[Image:Rote 58 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 59 || [[Image:Rote 59 Big.jpg|65px]] || 377 px <br> ↓ <br> 65 px | ||
| + | |- | ||
| + | | 60 || [[Image:Rote 60 Big.jpg|155px]] || 905 px <br> ↓ <br> 155 px | ||
| + | |- | ||
| + | | 64 || [[Image:Rote 64 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 81 || [[Image:Rote 81 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 105 || [[Image:Rote 105 Big.jpg|145px]] || 857 px <br> ↓ <br> 145 px | ||
| + | |- | ||
| + | | 128 || [[Image:Rote 128 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 165 || [[Image:Rote 165 Big.jpg|120px]] || 713 px <br> ↓ <br> 120 px | ||
| + | |- | ||
| + | | 256 || [[Image:Rote 256 Big.jpg|90px]] || 521 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 512 || [[Image:Rote 512 Big.jpg|105px]] || 617 px <br> ↓ <br> 105 px | ||
| + | |- | ||
| + | | 2010 || [[Image:Rote 2010 Big.jpg|190px]] || 1145 px <br> ↓ <br> 190 px | ||
| + | |- | ||
| + | | 2500 || [[Image:Rote 2500 Big.jpg|170px]] || 1001 px <br> ↓ <br> 170 px | ||
| + | |- | ||
| + | | 65536 || [[Image:Rote 65536 Big.jpg|115px]] || 665 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 802701 || [[Image:Rote 802701 Big.jpg|330px]] || 1961 px <br> ↓ <br> 330 px | ||
| + | |- | ||
| + | | 123456789 || [[Image:Rote 123456789 Big.jpg|345px]] || 2048 px <br> ↓ <br> 345 px | ||
| + | |} | ||
| + | |||
| + | ===Reduction 10:1=== | ||
| + | |||
| + | ====Cacti==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="50%" | '''Image''' | ||
| + | | width="50%" | '''Scale''' | ||
| + | |- | ||
| + | | [[Image:Rooted Node Big.jpg|12px]] || 117 px <br> ↓ <br> 12 px | ||
| + | |- | ||
| + | | [[Image:Rooted Edge Big.jpg|12px]] || 117 px <br> ↓ <br> 12 px | ||
| + | |} | ||
| + | |||
| + | ====Riffs==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="15%" | '''Integer''' | ||
| + | | width="70%" | '''Riff''' | ||
| + | | width="15%" | '''Scale''' | ||
| + | |- | ||
| + | | 1 || || | ||
| + | |- | ||
| + | | 2 || [[Image:Riff 2 Big.jpg|12px]] || 117 px <br> ↓ <br> 12 px | ||
| + | |- | ||
| + | | 3 || [[Image:Riff 3 Big.jpg|24px]] || 240 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 4 || [[Image:Riff 4 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 5 || [[Image:Riff 5 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 6 || [[Image:Riff 6 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 7 || [[Image:Riff 7 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 8 || [[Image:Riff 8 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 9 || [[Image:Riff 9 Big.jpg|24px]] || 240 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 10 || [[Image:Riff 10 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 11 || [[Image:Riff 11 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 12 || [[Image:Riff 12 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 13 || [[Image:Riff 13 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 14 || [[Image:Riff 14 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 15 || [[Image:Riff 15 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 16 || [[Image:Riff 16 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 17 || [[Image:Riff 17 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 18 || [[Image:Riff 18 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 19 || [[Image:Riff 19 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 20 || [[Image:Riff 20 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 21 || [[Image:Riff 21 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 22 || [[Image:Riff 22 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 23 || [[Image:Riff 23 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 24 || [[Image:Riff 24 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 25 || [[Image:Riff 25 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 26 || [[Image:Riff 26 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 27 || [[Image:Riff 27 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 28 || [[Image:Riff 28 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 29 || [[Image:Riff 29 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 30 || [[Image:Riff 30 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 31 || [[Image:Riff 31 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 32 || [[Image:Riff 32 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 33 || [[Image:Riff 33 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 34 || [[Image:Riff 34 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 35 || [[Image:Riff 35 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 36 || [[Image:Riff 36 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 37 || [[Image:Riff 37 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 38 || [[Image:Riff 38 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 39 || [[Image:Riff 39 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 40 || [[Image:Riff 40 Big.jpg|82px]] || 816 px <br> ↓ <br> 82 px | ||
| + | |- | ||
| + | | 41 || [[Image:Riff 41 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 42 || [[Image:Riff 42 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 43 || [[Image:Riff 43 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 44 || [[Image:Riff 44 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 45 || [[Image:Riff 45 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 46 || [[Image:Riff 46 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 47 || [[Image:Riff 47 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 48 || [[Image:Riff 48 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 49 || [[Image:Riff 49 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 50 || [[Image:Riff 50 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 51 || [[Image:Riff 51 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 52 || [[Image:Riff 52 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 53 || [[Image:Riff 53 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 54 || [[Image:Riff 54 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 55 || [[Image:Riff 55 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 56 || [[Image:Riff 56 Big.jpg|82px]] || 809 px <br> ↓ <br> 82 px | ||
| + | |- | ||
| + | | 57 || [[Image:Riff 57 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 58 || [[Image:Riff 58 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 59 || [[Image:Riff 59 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 60 || [[Image:Riff 60 Big.jpg|68px]] || 672 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 64 || [[Image:Riff 64 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 81 || [[Image:Riff 81 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 105 || [[Image:Riff 105 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 128 || [[Image:Riff 128 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 165 || [[Image:Riff 165 Big.jpg|82px]] || 816 px <br> ↓ <br> 82 px | ||
| + | |- | ||
| + | | 256 || [[Image:Riff 256 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 512 || [[Image:Riff 512 Big.jpg|38px]] || 384 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 2010 || [[Image:Riff 2010 Big.jpg|110px]] || 1104 px <br> ↓ <br> 110 px | ||
| + | |- | ||
| + | | 2500 || [[Image:Riff 2500 Big.jpg|54px]] || 528 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 65536 || [[Image:Riff 65536 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 802701 || [[Image:Riff 802701 Big.jpg|125px]] || 1248 px <br> ↓ <br> 125 px | ||
| + | |- | ||
| + | | 123456789 || [[Image:Riff 123456789 Big.jpg|130px]] || 1296 px <br> ↓ <br> 130 px | ||
| + | |} | ||
| + | |||
| + | ====Rotes==== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="20" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | width="15%" | '''Integer''' | ||
| + | | width="70%" | '''Rote''' | ||
| + | | width="15%" | '''Scale''' | ||
| + | |- | ||
| + | | 1 || [[Image:Rote 1 Big.jpg|12px]] || 117 px <br> ↓ <br> 12 px | ||
| + | |- | ||
| + | | 2 || [[Image:Rote 2 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 3 || [[Image:Rote 3 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 4 || [[Image:Rote 4 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 5 || [[Image:Rote 5 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 6 || [[Image:Rote 6 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 7 || [[Image:Rote 7 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 8 || [[Image:Rote 8 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 9 || [[Image:Rote 9 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 10 || [[Image:Rote 10 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 11 || [[Image:Rote 11 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 12 || [[Image:Rote 12 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 13 || [[Image:Rote 13 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 14 || [[Image:Rote 14 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 15 || [[Image:Rote 15 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 16 || [[Image:Rote 16 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 17 || [[Image:Rote 17 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 18 || [[Image:Rote 18 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 19 || [[Image:Rote 19 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 20 || [[Image:Rote 20 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 21 || [[Image:Rote 21 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 22 || [[Image:Rote 22 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 23 || [[Image:Rote 23 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 24 || [[Image:Rote 24 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 25 || [[Image:Rote 25 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 26 || [[Image:Rote 26 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 27 || [[Image:Rote 27 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 28 || [[Image:Rote 28 Big.jpg|76px]] || 761 px <br> ↓ <br> 76 px | ||
| + | |- | ||
| + | | 29 || [[Image:Rote 29 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 30 || [[Image:Rote 30 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 31 || [[Image:Rote 31 Big.jpg|24px]] || 233 px <br> ↓ <br> 24 px | ||
| + | |- | ||
| + | | 32 || [[Image:Rote 32 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 33 || [[Image:Rote 33 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 34 || [[Image:Rote 34 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 35 || [[Image:Rote 35 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 36 || [[Image:Rote 36 Big.jpg|86px]] || 857 px <br> ↓ <br> 86 px | ||
| + | |- | ||
| + | | 37 || [[Image:Rote 37 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 38 || [[Image:Rote 38 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 39 || [[Image:Rote 39 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 40 || [[Image:Rote 40 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 41 || [[Image:Rote 41 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 42 || [[Image:Rote 42 Big.jpg|86px]] || 857 px <br> ↓ <br> 86 px | ||
| + | |- | ||
| + | | 43 || [[Image:Rote 43 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 44 || [[Image:Rote 44 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 45 || [[Image:Rote 45 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 46 || [[Image:Rote 46 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 47 || [[Image:Rote 47 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 48 || [[Image:Rote 48 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 49 || [[Image:Rote 49 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 50 || [[Image:Rote 50 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 51 || [[Image:Rote 51 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 52 || [[Image:Rote 52 Big.jpg|86px]] || 857 px <br> ↓ <br> 86 px | ||
| + | |- | ||
| + | | 53 || [[Image:Rote 53 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 54 || [[Image:Rote 54 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 55 || [[Image:Rote 55 Big.jpg|48px]] || 473 px <br> ↓ <br> 48 px | ||
| + | |- | ||
| + | | 56 || [[Image:Rote 56 Big.jpg|76px]] || 761 px <br> ↓ <br> 76 px | ||
| + | |- | ||
| + | | 57 || [[Image:Rote 57 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 58 || [[Image:Rote 58 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 59 || [[Image:Rote 59 Big.jpg|38px]] || 377 px <br> ↓ <br> 38 px | ||
| + | |- | ||
| + | | 60 || [[Image:Rote 60 Big.jpg|90px]] || 905 px <br> ↓ <br> 90 px | ||
| + | |- | ||
| + | | 64 || [[Image:Rote 64 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 81 || [[Image:Rote 81 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 105 || [[Image:Rote 105 Big.jpg|86px]] || 857 px <br> ↓ <br> 86 px | ||
| + | |- | ||
| + | | 128 || [[Image:Rote 128 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 165 || [[Image:Rote 165 Big.jpg|72px]] || 713 px <br> ↓ <br> 72 px | ||
| + | |- | ||
| + | | 256 || [[Image:Rote 256 Big.jpg|54px]] || 521 px <br> ↓ <br> 54 px | ||
| + | |- | ||
| + | | 512 || [[Image:Rote 512 Big.jpg|62px]] || 617 px <br> ↓ <br> 62 px | ||
| + | |- | ||
| + | | 2010 || [[Image:Rote 2010 Big.jpg|115px]] || 1145 px <br> ↓ <br> 115 px | ||
| + | |- | ||
| + | | 2500 || [[Image:Rote 2500 Big.jpg|100px]] || 1001 px <br> ↓ <br> 100 px | ||
| + | |- | ||
| + | | 65536 || [[Image:Rote 65536 Big.jpg|68px]] || 665 px <br> ↓ <br> 68 px | ||
| + | |- | ||
| + | | 802701 || [[Image:Rote 802701 Big.jpg|196px]] || 1961 px <br> ↓ <br> 196 px | ||
| + | |- | ||
| + | | 123456789 || [[Image:Rote 123456789 Big.jpg|205px]] || 2048 px <br> ↓ <br> 205 px | ||
| + | |} | ||
| + | |||
| + | ==Riffs in Numerical Order== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="10" | ||
| + | |+ style="height:25px" | <math>\text{Riffs in Numerical Order}\!</math> | ||
| + | | valign="bottom" | | ||
| + | <p> </p><br> | ||
| + | <p><math>1\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} \varnothing \\ 1 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 2 Big.jpg|20px]]</p><br> | ||
| + | <p><math>\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 \\ 2 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 3 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 \\ 3 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 4 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 \\ 4 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 5 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 \\ 5 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 6 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 7 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 4\!:\!1 \\ 7 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 8 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 \\ 8 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 9 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!2 \\ 9 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 10 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 11 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 5\!:\!1 \\ 11 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 12 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 13 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 6\!:\!1 \\ 13 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 14 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 15 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 16 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!4 \\ 16 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 17 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 7\!:\!1 \\ 17 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 18 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 19 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 8\!:\!1 \\ 19 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 20 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 21 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 22 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 23 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 9\!:\!1 \\ 23 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 24 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 25 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!2 \\ 25 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 26 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 27 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!3 \\ 27 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 28 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 29 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 10\!:\!1 \\ 29 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 30 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 31 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 11\!:\!1 \\ 31 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 32 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!5 \\ 32 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 33 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 34 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 35 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 36 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 37 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 12\!:\!1 \\ 37 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 38 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 39 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 40 Big.jpg|135px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 41 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 13\!:\!1 \\ 41 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 42 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 43 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 14\!:\!1 \\ 43 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 44 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 45 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 46 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 47 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 15\!:\!1 \\ 47 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 48 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 49 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 4\!:\!2 \\ 49 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 50 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 51 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 52 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 53 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 16\!:\!1 \\ 53 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 54 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 55 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 56 Big.jpg|135px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 57 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 58 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 59 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 17\!:\!1 \\ 59 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Riff 60 Big.jpg|115px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}</math></p> | ||
| + | |} | ||
| + | |||
| + | ==Rotes in Numerical Order== | ||
| + | |||
| + | {| align="center" border="1" cellpadding="6" | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 1 Big.jpg|20px]]</p><br> | ||
| + | <p><math>1\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} \varnothing \\ 1 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 2 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 \\ 2 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 3 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 \\ 3 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 4 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 \\ 4 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 5 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 \\ 5 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 6 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 7 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 4\!:\!1 \\ 7 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 8 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 \\ 8 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 9 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!2 \\ 9 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 10 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 11 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 5\!:\!1 \\ 11 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 12 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 13 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 6\!:\!1 \\ 13 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 14 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 15 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 16 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!4 \\ 16 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 17 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 7\!:\!1 \\ 17 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 18 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 19 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 8\!:\!1 \\ 19 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 20 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 21 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 22 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 23 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 9\!:\!1 \\ 23 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 24 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 25 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!2 \\ 25 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 26 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 27 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!3 \\ 27 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 28 Big.jpg|130px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 29 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 10\!:\!1 \\ 29 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 30 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 31 Big.jpg|40px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 11\!:\!1 \\ 31 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 32 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!5 \\ 32 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 33 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 34 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 35 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 36 Big.jpg|145px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 37 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 12\!:\!1 \\ 37 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 38 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 39 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 40 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 41 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 13\!:\!1 \\ 41 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 42 Big.jpg|145px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 43 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 14\!:\!1 \\ 43 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 44 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 45 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 46 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 47 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 15\!:\!1 \\ 47 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 48 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 49 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 4\!:\!2 \\ 49 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 50 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 51 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 52 Big.jpg|145px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 53 Big.jpg|90px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}^{\text{p}^\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 16\!:\!1 \\ 53 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 54 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 55 Big.jpg|80px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}</math></p> | ||
| + | |- | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 56 Big.jpg|130px]]</p><br> | ||
| + | <p><math>\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 57 Big.jpg|105px]]</p><br> | ||
| + | <p><math>\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 58 Big.jpg|120px]]</p><br> | ||
| + | <p><math>\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 59 Big.jpg|65px]]</p><br> | ||
| + | <p><math>\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 17\!:\!1 \\ 59 \end{array}</math></p> | ||
| + | | valign="bottom" | | ||
| + | <p>[[Image:Rote 60 Big.jpg|155px]]</p><br> | ||
| + | <p><math>\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!</math></p><br> | ||
| + | <p><math>\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}</math></p> | ||
| + | |} | ||
| + | |||
| + | ==Miscellaneous Examples (Reduction 8:1)== | ||
| + | |||
| + | {| align="center" border="1" width="96%" | ||
| + | |+ style="height:24px" | <math>\text{Integers, Riffs, Rotes}\!</math> | ||
| + | |- style="height:50px; background:#f0f0ff" | ||
| + | | | ||
| + | {| cellpadding="12" style="background:#f0f0ff; text-align:center; width:100%" | ||
| + | | width="10%" | <math>\text{Integer}\!</math> | ||
| + | | width="45%" | <math>\text{Riff}\!</math> | ||
| + | | width="45%" | <math>\text{Rote}\!</math> | ||
| + | |} | ||
| + | |- | ||
| + | | | ||
| + | {| cellpadding="12" style="text-align:center; width:100%" | ||
| + | | width="10%" | <math>1\!</math> | ||
| + | | width="45%" | | ||
| + | | width="45%" | [[Image:Rote 1 Big.jpg|15px]] | ||
| + | |- | ||
| + | | <math>2\!</math> | ||
| + | | [[Image:Riff 2 Big.jpg|15px]] | ||
| + | | [[Image:Rote 2 Big.jpg|30px]] | ||
| + | |- | ||
| + | | <math>3\!</math> | ||
| + | | [[Image:Riff 3 Big.jpg|30px]] | ||
| + | | [[Image:Rote 3 Big.jpg|30px]] | ||
| + | |- | ||
| + | | <math>4\!</math> | ||
| + | | [[Image:Riff 4 Big.jpg|30px]] | ||
| + | | [[Image:Rote 4 Big.jpg|48px]] | ||
| + | |- | ||
| + | | <math>2010\!</math> | ||
| + | | [[Image:Riff 2010 Big.jpg|138px]] | ||
| + | | [[Image:Rote 2010 Big.jpg|144px]] | ||
| + | |- | ||
| + | | <math>2011\!</math> | ||
| + | | [[Image:Riff 2011 Big.jpg|84px]] | ||
| + | | [[Image:Rote 2011 Big.jpg|120px]] | ||
| + | |- | ||
| + | | <math>2500\!</math> | ||
| + | | [[Image:Riff 2500 Big.jpg|66px]] | ||
| + | | [[Image:Rote 2500 Big.jpg|125px]] | ||
| + | |- | ||
| + | | <math>802701\!</math> | ||
| + | | [[Image:Riff 802701 Big.jpg|156px]] | ||
| + | | [[Image:Rote 802701 Big.jpg|245px]] | ||
| + | |- | ||
| + | | <math>123456789\!</math> | ||
| + | | [[Image:Riff 123456789 Big.jpg|162px]] | ||
| + | | [[Image:Rote 123456789 Big.jpg|256px]] | ||
| + | |} | ||
| + | |} | ||
| + | |||
| + | ==Cactus Graphs== | ||
| + | |||
| + | ===Hi Res=== | ||
| + | |||
| + | <br> | ||
| + | |||
| + | {| align="center" border="1" cellpadding="8" cellspacing="0" style="text-align:center; width:90%" | ||
| + | |- | ||
| + | | height="100px" | [[Image:Rooted Node Big.jpg|20px]] | ||
| + | | 117 px → 20 px | ||
| + | |- | ||
| + | | height="100px" | [[Image:Rooted Edge Big.jpg|20px]] | ||
| + | | 117 px → 20 px | ||
| + | |- | ||
| + | | height="100px" | [[Image:Cactus A Big.jpg|20px]] | ||
| + | | 117 px → 20 px | ||
| + | |- | ||
| + | | height="120px" | [[Image:Cactus (A) Big.jpg|20px]] | ||
| + | | 117 px → 20 px | ||
| + | |- | ||
| + | | height="100px" | [[Image:Cactus ABC Big.jpg|50px]] | ||
| + | | 290 px → 50 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus ((A)(B)(C)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="120px" | [[Image:Cactus (A)B Big.jpg|35px]] | ||
| + | | 204 px → 35 px | ||
| + | |- | ||
| + | | height="120px" | [[Image:Cactus (A(B)) Big.jpg|60px]] | ||
| + | | 348 px → 60 px | ||
| + | |- | ||
| + | | height="120px" | [[Image:Cactus (A,B) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus ((A,B)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="120px" | [[Image:Cactus (A,B,C) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus ((A),(B),(C)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus ((A,B,C)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="200px" | [[Image:Cactus (((A),(B),(C))) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus (A,(B),(C)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="200px" | [[Image:Cactus (((A),B,C)) Big.jpg|65px]] | ||
| + | | 386 px → 65 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus (A,(B,C)) Big.jpg|90px]] | ||
| + | | 530 px → 90 px | ||
| + | |- | ||
| + | | height="160px" | [[Image:Cactus (X,(A),(B),(C)) Big.jpg|90px]] | ||
| + | | 530 px → 90 px | ||
| + | |} | ||
| + | |||
| + | <br> | ||
| + | |||
| + | ===Lo Res=== | ||
| + | |||
| + | <br> | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | [[Image:Cactus Graph Node Connective.jpg]] | ||
| + | |- | ||
| + | | [[Image:Cactus Graph Lobe Connective.jpg]] | ||
| + | |- | ||
| + | | [[Image:Cactus Graph Lobe Rule.jpg]] | ||
| + | |- | ||
| + | | [[Image:Cactus Graph Spike Rule.jpg]] | ||
| + | |} | ||
| + | |||
| + | <br> | ||
| + | |||
==Differential Logic== | ==Differential Logic== | ||
===ASCII Graphics=== | ===ASCII Graphics=== | ||
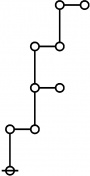
| − | {| align="center" | + | ====Series 1==== |
| + | |||
| + | {| align="center" cellpadding="10" style="text-align:center; width:90%" | ||
| | | | ||
<pre> | <pre> | ||
| Line 33: | Line 1,337: | ||
|} | |} | ||
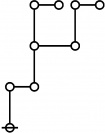
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center; width:90%" |
| | | | ||
<pre> | <pre> | ||
| Line 77: | Line 1,381: | ||
|} | |} | ||
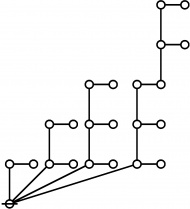
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center; width:90%" |
| | | | ||
<pre> | <pre> | ||
| Line 121: | Line 1,425: | ||
|} | |} | ||
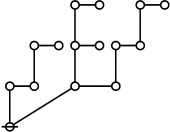
| − | {| align="center" | + | {| align="center" cellpadding="10" style="text-align:center; width:90%" |
| | | | ||
<pre> | <pre> | ||
| Line 158: | Line 1,462: | ||
</pre> | </pre> | ||
|} | |} | ||
| + | |||
| + | ====Series 2==== | ||
{| align="center" cellspacing="10" style="text-align:center; width:90%" | {| align="center" cellspacing="10" style="text-align:center; width:90%" | ||
| Line 421: | Line 1,727: | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
\operatorname{E}(pq) | \operatorname{E}(pq) | ||
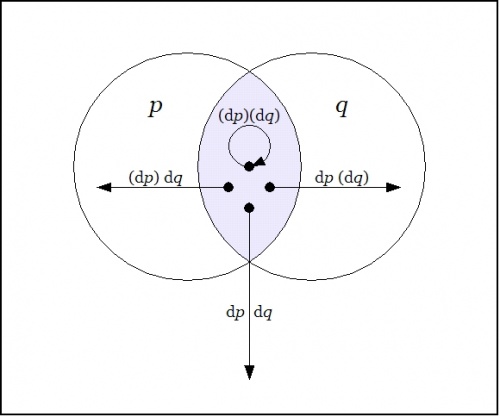
| − | & = & | + | & = & |
| + | p | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & | + | & + & |
| + | p | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & (q) & \cdot & ~\operatorname{d}p~~\operatorname{d}q~ | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\end{array}</math> | \end{array}</math> | ||
|} | |} | ||
| Line 439: | Line 1,769: | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
\operatorname{D}(pq) | \operatorname{D}(pq) | ||
| − | & = & | + | & = & |
| + | p | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{(} | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
| + | \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & | + | & + & |
| + | p | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
| + | \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
| + | \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & (q) & \cdot & ~~\operatorname{d}p~~\operatorname{d}q~~ | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(}q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
| + | \texttt{~} | ||
\end{array}</math> | \end{array}</math> | ||
|} | |} | ||
| Line 465: | Line 1,827: | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
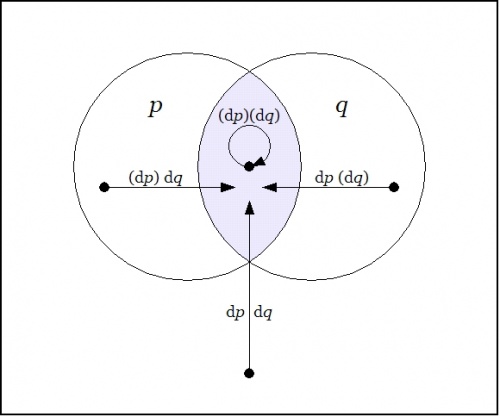
\varepsilon (pq) | \varepsilon (pq) | ||
| − | & = & p & \cdot & q & \cdot & (\operatorname{d}p)(\operatorname{d}q) | + | & = & |
| + | p & \cdot & q & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & p & \cdot & q & \cdot & (\operatorname{d}p)~\operatorname{d}q~ | + | & + & |
| + | p & \cdot & q & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & p & \cdot & q & \cdot & ~\operatorname{d}p~(\operatorname{d}q) | + | & + & |
| + | p & \cdot & q & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & p & \cdot & q & \cdot & ~\operatorname{d}p~~\operatorname{d}q~ | + | & + & |
| + | p & \cdot & q & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\end{array}</math> | \end{array}</math> | ||
|} | |} | ||
| Line 483: | Line 1,857: | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
\operatorname{E}(pq) | \operatorname{E}(pq) | ||
| − | & = & | + | & = & |
| + | p | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & | + | & + & |
| + | p | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & (q) & \cdot & ~\operatorname{d}p~~\operatorname{d}q~ | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
\end{array}</math> | \end{array}</math> | ||
|} | |} | ||
| Line 501: | Line 1,899: | ||
<math>\begin{array}{rcccccc} | <math>\begin{array}{rcccccc} | ||
\operatorname{D}(pq) | \operatorname{D}(pq) | ||
| − | & = & | + | & = & |
| + | p | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{(} | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
| + | \texttt{)} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & | + | & + & |
| + | p | ||
| + | & \cdot & | ||
| + | \texttt{(} q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{(} \operatorname{d}p \texttt{)} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
| + | \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | q | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{(} \operatorname{d}q \texttt{)} | ||
| + | \texttt{~} | ||
\\[4pt] | \\[4pt] | ||
| − | & + & (p) & \cdot & (q) & \cdot & ~~\operatorname{d}p~~\operatorname{d}q~~ | + | & + & |
| + | \texttt{(} p \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{(}q \texttt{)} | ||
| + | & \cdot & | ||
| + | \texttt{~} | ||
| + | \texttt{~} \operatorname{d}p \texttt{~} | ||
| + | \texttt{~} \operatorname{d}q \texttt{~} | ||
| + | \texttt{~} | ||
\end{array}</math> | \end{array}</math> | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellspacing="10" style="text-align:center" | ||
| + | | [[Image:Field Picture PQ Differential Conjunction.jpg|500px]] | ||
| + | |- | ||
| + | | <math>\text{Figure 26-1. Tangent Map}~ \operatorname{d}(pq) : \operatorname{E}X \to \mathbb{B}</math> | ||
| + | |- | ||
| + | | | ||
| + | <math>\begin{array}{rcccccc} | ||
| + | \operatorname{d}(pq) | ||
| + | & = & | ||
| + | p & \cdot & q & \cdot & | ||
| + | \texttt{(} \operatorname{d}p \texttt{,} \operatorname{d}q \texttt{)} | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | p & \cdot & \texttt{(} q \texttt{)} & \cdot & | ||
| + | \operatorname{d}q | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | \texttt{(} p \texttt{)} & \cdot & q & \cdot & | ||
| + | \operatorname{d}p | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | \texttt{(} p \texttt{)} & \cdot & \texttt{(} q \texttt{)} & \cdot & 0 | ||
| + | \end{array}</math> | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellspacing="10" style="text-align:center" | ||
| + | | [[Image:Field Picture PQ Remainder Conjunction.jpg|500px]] | ||
| + | |- | ||
| + | | <math>\text{Figure 26-2. Remainder Map}~ \operatorname{r}(pq) : \operatorname{E}X \to \mathbb{B}</math> | ||
| + | |- | ||
| + | | | ||
| + | <math>\begin{array}{rcccccc} | ||
| + | \operatorname{r}(pq) | ||
| + | & = & | ||
| + | p & \cdot & q & \cdot & | ||
| + | \operatorname{d}p ~ \operatorname{d}q | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | p & \cdot & \texttt{(} q \texttt{)} & \cdot & | ||
| + | \operatorname{d}p ~ \operatorname{d}q | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | \texttt{(} p \texttt{)} & \cdot & q & \cdot & | ||
| + | \operatorname{d}p ~ \operatorname{d}q | ||
| + | \\[4pt] | ||
| + | & + & | ||
| + | \texttt{(} p \texttt{)} & \cdot & \texttt{(} q \texttt{)} & \cdot & | ||
| + | \operatorname{d}p ~ \operatorname{d}q | ||
| + | \end{array}</math> | ||
| + | |} | ||
| + | |||
| + | ==Propositional Equation Reasoning Systems== | ||
| + | |||
| + | ===Analysis of contingent propositions=== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | [[Image:Logical Graph (P (Q)) (P (R)).jpg|500px]] || (26) | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellpadding="8" style="text-align:center" | ||
| + | | [[Image:Venn Diagram (P (Q)) (P (R)).jpg|500px]] || (27) | ||
| + | |- | ||
| + | | <math>\text{Venn Diagram for}~ \texttt{(} p \texttt{~(} q \texttt{))~(} p \texttt{~(} r \texttt{))}</math> | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellpadding="8" style="text-align:center" | ||
| + | | [[Image:Venn Diagram (P (Q R)).jpg|500px]] || (28) | ||
| + | |- | ||
| + | | <math>\text{Venn Diagram for}~ \texttt{(} p \texttt{~(} q ~ r \texttt{))}</math> | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | [[Image:Logical Graph (P (Q)) (P (R)) = (P (Q R)).jpg|500px]] || (29) | ||
| + | |} | ||
| + | |||
| + | ====Equation 1 : Proof 1==== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | [[Image:Logical Graph (P (Q)) (P (R)) = (P (Q R)) Proof 1.jpg|500px]] | ||
| + | | (30) | ||
| + | |} | ||
| + | |||
| + | ====Equation 1 : Proof 2==== | ||
| + | |||
| + | =====Single Image Version===== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | [[Image:Logical Graph (P (Q)) (P (R)) = (P (Q R)) Proof 2a Alt.jpg|500px]] | ||
| + | | (31) | ||
| + | |} | ||
| + | |||
| + | =====Serial Image Version===== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | | ||
| + | {| align="center" cellpadding="0" cellspacing="0" style="border-left:1px solid black; border-top:1px solid black; border-right:1px solid black; border-bottom:1px solid black; text-align:center" | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-0.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-1.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast P.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-2.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-3.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-4.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast Q.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-5.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-6.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-7.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast R.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-8.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-1-9.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- DNF.jpg|500px]] | ||
| + | |} | ||
| + | | (31) | ||
| + | |} | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | | ||
| + | {| align="center" cellpadding="0" cellspacing="0" style="border-left:1px solid black; border-top:1px solid black; border-right:1px solid black; border-bottom:1px solid black; text-align:center" | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-0.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-1.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast P.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-2.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-3.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-4.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast Q.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-5.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-6.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-7.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast R.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-8.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 2-2-9.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- DNF.jpg|500px]] | ||
| + | |} | ||
| + | | (33) | ||
| + | |} | ||
| + | |||
| + | ====Equation 1 : Proof 3==== | ||
| + | |||
| + | {| | ||
| + | | | ||
| + | * '''Variant 1''' | ||
| + | # start | ||
| + | # cast p | ||
| + | # dom | ||
| + | # can | ||
| + | # empty | ||
| + | # can | ||
| + | # cast q | ||
| + | # dom | ||
| + | # can | ||
| + | # dom | ||
| + | # spike | ||
| + | # can | ||
| + | # cast r | ||
| + | # can | ||
| + | # empty | ||
| + | # spike | ||
| + | # can | ||
| + | | | ||
| + | * '''Variant 2''' | ||
| + | # start | ||
| + | # cast p | ||
| + | # dom | ||
| + | # can | ||
| + | # empty | ||
| + | # can | ||
| + | # cast q | ||
| + | # can | ||
| + | # dom | ||
| + | # can | ||
| + | # spike | ||
| + | # can | ||
| + | # cast r | ||
| + | # can | ||
| + | # empty | ||
| + | # spike | ||
| + | # can | ||
| + | |} | ||
| + | |||
| + | =====Variant 1===== | ||
| + | |||
| + | {| align="center" cellpadding="8" style="text-align:center; width:90%" | ||
| + | | | ||
| + | <pre> | ||
| + | o-----------------------------------------------------------o | ||
| + | | Equation E_1. Proof 3. | | ||
| + | o-----------------------------------------------------------o | ||
| + | | 1 | | ||
| + | | q o o r q o r | | ||
| + | | | | | | | ||
| + | | p o o p p o | | ||
| + | | \ / | | | ||
| + | | o---------o | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | o | | ||
| + | | | | | ||
| + | | | | | ||
| + | | | | | ||
| + | | | | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< CAST "p" >=============o | ||
| + | | 2 | | ||
| + | | q r q r q r qr | | ||
| + | | o o o o o o o o o | | ||
| + | | | | | |/ |/ |/ | | ||
| + | | o o o o o o | | ||
| + | | \ / | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | p o---------------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Domination >===========o | ||
| + | | 3 | | ||
| + | | q r q r | | ||
| + | | o o o o o o | | ||
| + | | | | | / / / | | ||
| + | | o o o o o o | | ||
| + | | \ / | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | p o---------------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 4 | | ||
| + | | q r q r | | ||
| + | | o o o | | ||
| + | | | | | | | ||
| + | | o o o | | ||
| + | | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | p o---------------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Emptiness >============o | ||
| + | | 5 | | ||
| + | | q r q r | | ||
| + | | o o o | | ||
| + | | | | | | | ||
| + | | o o o | | ||
| + | | \ / | | | ||
| + | | o-------o o | | ||
| + | | \ / | | | ||
| + | | \ / | | | ||
| + | | \ / | | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | p o---------------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 6 | | ||
| + | | q r q r | | ||
| + | | o o o | | ||
| + | | | | | | | ||
| + | | o o o | | ||
| + | | \ / | | | ||
| + | | o-------o | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | o | | ||
| + | | | | | ||
| + | | | | | ||
| + | | | | | ||
| + | | p o---------------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< CAST "q" >=============o | ||
| + | | 7 | | ||
| + | | o o | | ||
| + | | r r | r | | | ||
| + | | o o o o o o r | | ||
| + | | | | | | | | | | ||
| + | | o o o o o o | | ||
| + | | \ / | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Domination >===========o | ||
| + | | 8 | | ||
| + | | o o | | ||
| + | | r r | r | | | ||
| + | | o o o o o o | | ||
| + | | | | | | | | | | ||
| + | | o o o o o o | | ||
| + | | \ / | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 9 | | ||
| + | | r r r | | ||
| + | | o o o | | ||
| + | | | | | | | ||
| + | | o o o o o | | ||
| + | | / | \ / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Domination >===========o | ||
| + | | 10 | | ||
| + | | r r | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | o o o o | | ||
| + | | / | \ | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Spike >================o | ||
| + | | 11 | | ||
| + | | r r | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | o o | | ||
| + | | / | | | ||
| + | | o-------o o | | ||
| + | | \ / | | | ||
| + | | \ / | | | ||
| + | | \ / | | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 12 | | ||
| + | | r r | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | o o | | ||
| + | | / | | | ||
| + | | o-------o | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | o | | ||
| + | | | | | ||
| + | | | | | ||
| + | | | | | ||
| + | | q o---------------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< CAST "r" >=============o | ||
| + | | 13 | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | o o o o | | ||
| + | | | | | | | | ||
| + | | o o o o | | ||
| + | | / | / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | r o---------------o---o r | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | q o-------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 14 | | ||
| + | | o o | | ||
| + | | / | | | ||
| + | | o-------o o-------o | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | \ / \ / | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | r o---------------o---o r | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | q o-------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Emptiness & Spike >====o | ||
| + | | 15 | | ||
| + | | o o 16 | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | o o | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | | | | | ||
| + | | r o---------------o---o r | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | q o-------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< Cancellation >=========o | ||
| + | | 17 | | ||
| + | | r o-------o---o r | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | q o-------o---o q | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | p o-------o---o p | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | \ / | | ||
| + | | @ | | ||
| + | | | | ||
| + | o==================================< QED >==================o | ||
| + | </pre> | ||
| + | | (40) | ||
| + | |} | ||
| + | |||
| + | =====Variant 2===== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | | ||
| + | {| align="center" cellpadding="0" cellspacing="0" style="border-left:1px solid black; border-top:1px solid black; border-right:1px solid black; border-bottom:1px solid black; text-align:center" | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-00.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-01.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast P.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-02.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-03.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-04.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Emptiness.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-05.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-06.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast Q.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-07.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-08.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-09.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-10.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Spike.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-11.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-12.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast R.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-13.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-14.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Emptiness.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-15.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Spike.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-16.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof (P (Q)) (P (R)) = (P (Q R)) 3-17.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- QED.jpg|500px]] | ||
| + | |} | ||
| + | | (40) | ||
| + | |} | ||
| + | |||
| + | ===Praeclarum Theorema : Proof by CAST=== | ||
| + | |||
| + | {| align="center" cellpadding="8" | ||
| + | | | ||
| + | {| align="center" cellpadding="0" cellspacing="0" style="border-left:1px solid black; border-top:1px solid black; border-right:1px solid black; border-bottom:1px solid black; text-align:center" | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 00.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 01.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast A.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 02.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 03.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 04.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 05.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 06.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast D.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 07.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 08.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 09.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 10.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 11.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast B.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 12.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 13.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Domination.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 14.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 15.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cast C.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 16.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 17.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- Cancellation.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Proof Praeclarum Theorema CAST 18.jpg|500px]] | ||
| + | |- | ||
| + | | [[Image:Equational Inference Bar -- QED.jpg|500px]] | ||
| + | |} | ||
| + | | (23) | ||
|} | |} | ||
Latest revision as of 18:52, 7 January 2011
Animations
Riffs 1 to 60

|
Rotes 1 to 60

|
Image Gallery
Reduction 6:1
Cacti
| Image | Scale |
| 117 px ↓ 20 px | |
| 117 px ↓ 20 px |
Riffs
Rotes
Reduction 10:1
Cacti
| Image | Scale |
| 117 px ↓ 12 px | |
| 117 px ↓ 12 px |
Riffs
Rotes
Riffs in Numerical Order
|
\(1\!\) \(\begin{array}{l} \varnothing \\ 1 \end{array}\) |
\(\text{p}\!\) \(\begin{array}{l} 1\!:\!1 \\ 2 \end{array}\) |
\(\text{p}_\text{p}\!\) \(\begin{array}{l} 2\!:\!1 \\ 3 \end{array}\) |
\(\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 \\ 4 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 \\ 5 \end{array}\) |
|
\(\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 4\!:\!1 \\ 7 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 \\ 8 \end{array}\) |
\(\text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 2\!:\!2 \\ 9 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 5\!:\!1 \\ 11 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 6\!:\!1 \\ 13 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}\) |
|
\(\text{p}^{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!4 \\ 16 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 7\!:\!1 \\ 17 \end{array}\) |
\(\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 8\!:\!1 \\ 19 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 9\!:\!1 \\ 23 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 3\!:\!2 \\ 25 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}\) |
\(\text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!3 \\ 27 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 10\!:\!1 \\ 29 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!\) \(\begin{array}{l} 11\!:\!1 \\ 31 \end{array}\) |
\(\text{p}^{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!5 \\ 32 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}\) |
|
\(\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}\) |
\(\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 12\!:\!1 \\ 37 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!\) \(\begin{array}{l} 13\!:\!1 \\ 41 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 14\!:\!1 \\ 43 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}\) |
\(\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}\) |
\(\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 15\!:\!1 \\ 47 \end{array}\) |
\(\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}^\text{p}\!\) \(\begin{array}{l} 4\!:\!2 \\ 49 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 16\!:\!1 \\ 53 \end{array}\) |
\(\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}\) |
|
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}\) |
\(\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!\) \(\begin{array}{l} 17\!:\!1 \\ 59 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}\) |
Rotes in Numerical Order
|
\(1\!\) \(\begin{array}{l} \varnothing \\ 1 \end{array}\) |
\(\text{p}\!\) \(\begin{array}{l} 1\!:\!1 \\ 2 \end{array}\) |
\(\text{p}_\text{p}\!\) \(\begin{array}{l} 2\!:\!1 \\ 3 \end{array}\) |
\(\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 \\ 4 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 \\ 5 \end{array}\) |
|
\(\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 \\ 6 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 4\!:\!1 \\ 7 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 \\ 8 \end{array}\) |
\(\text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 2\!:\!2 \\ 9 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!1 \\ 10 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 5\!:\!1 \\ 11 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 \\ 12 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 6\!:\!1 \\ 13 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 4\!:\!1 \\ 14 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 3\!:\!1 \\ 15 \end{array}\) |
|
\(\text{p}^{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!4 \\ 16 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 7\!:\!1 \\ 17 \end{array}\) |
\(\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!2 \\ 18 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 8\!:\!1 \\ 19 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 3\!:\!1 \\ 20 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 4\!:\!1 \\ 21 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 5\!:\!1 \\ 22 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 9\!:\!1 \\ 23 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 2\!:\!1 \\ 24 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 3\!:\!2 \\ 25 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 6\!:\!1 \\ 26 \end{array}\) |
\(\text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!3 \\ 27 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 4\!:\!1 \\ 28 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 10\!:\!1 \\ 29 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 30 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p}_{\text{p}_\text{p}}}}\!\) \(\begin{array}{l} 11\!:\!1 \\ 31 \end{array}\) |
\(\text{p}^{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!5 \\ 32 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 5\!:\!1 \\ 33 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 7\!:\!1 \\ 34 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 4\!:\!1 \\ 35 \end{array}\) |
|
\(\text{p}^\text{p} \text{p}_\text{p}^\text{p}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!2 \\ 36 \end{array}\) |
\(\text{p}_{\text{p}^\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 12\!:\!1 \\ 37 \end{array}\) |
\(\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 8\!:\!1 \\ 38 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 6\!:\!1 \\ 39 \end{array}\) |
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 3\!:\!1 \\ 40 \end{array}\) |
|
\(\text{p}_{\text{p}_{\text{p} \text{p}_\text{p}}}\!\) \(\begin{array}{l} 13\!:\!1 \\ 41 \end{array}\) |
\(\text{p} \text{p}_\text{p} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!1 ~~ 4\!:\!1 \\ 42 \end{array}\) |
\(\text{p}_{\text{p} \text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 14\!:\!1 \\ 43 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 5\!:\!1 \\ 44 \end{array}\) |
\(\text{p}_\text{p}^\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 2\!:\!2 ~~ 3\!:\!1 \\ 45 \end{array}\) |
|
\(\text{p} \text{p}_{\text{p}_\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 9\!:\!1 \\ 46 \end{array}\) |
\(\text{p}_{\text{p}_\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 15\!:\!1 \\ 47 \end{array}\) |
\(\text{p}^{\text{p}^\text{p}} \text{p}_\text{p}\!\) \(\begin{array}{l} 1\!:\!4 ~~ 2\!:\!1 \\ 48 \end{array}\) |
\(\text{p}_{\text{p}^\text{p}}^\text{p}\!\) \(\begin{array}{l} 4\!:\!2 \\ 49 \end{array}\) |
\(\text{p} \text{p}_{\text{p}_\text{p}}^\text{p}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 3\!:\!2 \\ 50 \end{array}\) |
|
\(\text{p}_\text{p} \text{p}_{\text{p}_{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 7\!:\!1 \\ 51 \end{array}\) |
\(\text{p}^\text{p} \text{p}_{\text{p} \text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 6\!:\!1 \\ 52 \end{array}\) |
\(\text{p}_{\text{p}^{\text{p}^\text{p}}}\!\) \(\begin{array}{l} 16\!:\!1 \\ 53 \end{array}\) |
\(\text{p} \text{p}_\text{p}^{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 2\!:\!3 \\ 54 \end{array}\) |
\(\text{p}_{\text{p}_\text{p}} \text{p}_{\text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 3\!:\!1 ~~ 5\!:\!1 \\ 55 \end{array}\) |
|
\(\text{p}^{\text{p}_\text{p}} \text{p}_{\text{p}^\text{p}}\!\) \(\begin{array}{l} 1\!:\!3 ~~ 4\!:\!1 \\ 56 \end{array}\) |
\(\text{p}_\text{p} \text{p}_{\text{p}^{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 2\!:\!1 ~~ 8\!:\!1 \\ 57 \end{array}\) |
\(\text{p} \text{p}_{\text{p} \text{p}_{\text{p}_\text{p}}}\!\) \(\begin{array}{l} 1\!:\!1 ~~ 10\!:\!1 \\ 58 \end{array}\) |
\(\text{p}_{\text{p}_{\text{p}_{\text{p}^\text{p}}}}\!\) \(\begin{array}{l} 17\!:\!1 \\ 59 \end{array}\) |
\(\text{p}^\text{p} \text{p}_\text{p} \text{p}_{\text{p}_\text{p}}\!\) \(\begin{array}{l} 1\!:\!2 ~~ 2\!:\!1 ~~ 3\!:\!1 \\ 60 \end{array}\) |
Miscellaneous Examples (Reduction 8:1)
| |||||||||||||||||||||||||||
|
Cactus Graphs
Hi Res
Lo Res
|
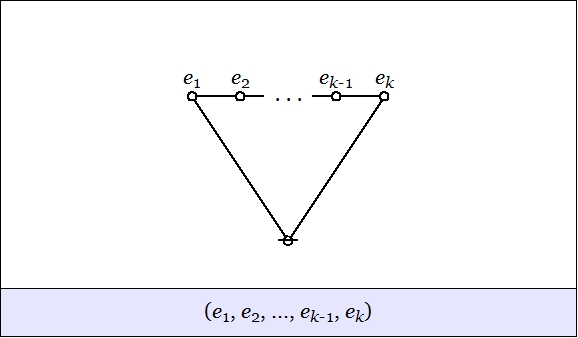
|
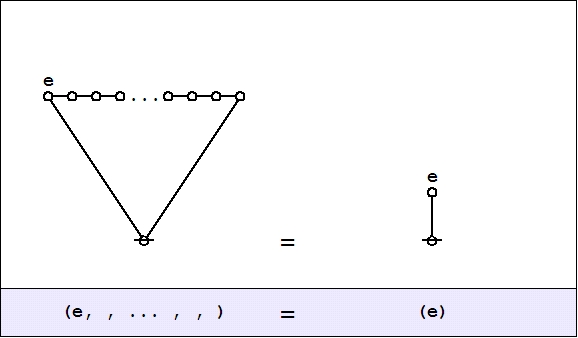
|

|
Differential Logic
ASCII Graphics
Series 1
o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / o \ | | / /%\ \ | | / /%%%\ \ | | o o%%%%%o o | | | |%%%%%| | | | | P |%%%%%| Q | | | | |%%%%%| | | | o o%%%%%o o | | \ \%%%/ / | | \ \%/ / | | \ o / | | \ / \ / | | o-------------o o-------------o | | | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o Figure 22-a. Conjunction pq : X -> B |
o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / P o Q \ | | / /%\ \ | | / /%%%\ \ | | o o.->-.o o | | | p(q)(dp)dq |%\%/%| (p)q dp(dq) | | | | o---------------|->o<-|---------------o | | | | |%%^%%| | | | o o%%|%%o o | | \ \%|%/ / | | \ \|/ / | | \ o / | | \ /|\ / | | o-------------o | o-------------o | | | | | | | | | | | o | | (p)(q) dp dq | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o | | | Ef = p q (dp)(dq) | | | | + p (q) (dp) dq | | | | + (p) q dp (dq) | | | | + (p)(q) dp dq | | | o-------------------------------------------------o Figure 22-b. Enlargement E[pq] : EX -> B |
o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / P o Q \ | | / /%\ \ | | / /%%%\ \ | | o o%%%%%o o | | | (dp)dq |%%%%%| dp(dq) | | | | o<--------------|->o<-|-------------->o | | | | |%%^%%| | | | o o%%|%%o o | | \ \%|%/ / | | \ \|/ / | | \ o / | | \ /|\ / | | o-------------o | o-------------o | | | | | | | | v | | o | | dp dq | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o | | | Df = p q ((dp)(dq)) | | | | + p (q) (dp) dq | | | | + (p) q dp (dq) | | | | + (p)(q) dp dq | | | o-------------------------------------------------o Figure 22-c. Difference D[pq] : EX -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o | | / \ | | / \ | | / \ | | / \ | | / \ | | / \ | | / \ | | o o | | | | | | | | | | | | | | | G | | | | | | | | | | | | | | | o o | | \ / | | \ / | | \ T / | | \ o<------------/-------------o | | \ / | | \ / | | \ / | | o-------------------o | | | | | o---------------------------------------------------------------------o Figure 23. Elements of a Cybernetic System |
Series 2
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / o \ | | / /%\ \ | | / /%%%\ \ | | / /%%%%%\ \ | | / /%%%%%%%\ \ | | / /%%%%%%%%%\ \ | | o o%%%%%%%%%%%o o | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | P |%%%%%%%%%%%| Q | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | o o%%%%%%%%%%%o o | | \ \%%%%%%%%%/ / | | \ \%%%%%%%/ / | | \ \%%%%%/ / | | \ \%%%/ / | | \ \%/ / | | \ o / | | \ / \ / | | o-------------------o o-------------------o | | | | | o---------------------------------------------------------------------o Figure 24-1. Proposition pq : X -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o (dp) (dq) o o | | | | o-->--o | | | | | | \ / | | | | | (dp) dq | \ / | dp (dq) | | | | o<-----------------o----------------->o | | | | | | | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 24-2. Tacit Extension !e![pq] : EX -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o (dp) (dq) o o | | | | o-->--o | | | | | | \ / | | | | | (dp) dq | \ / | dp (dq) | | | | o----------------->o<-----------------o | | | | | ^ | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | | | | o | | | o---------------------------------------------------------------------o Figure 25-1. Enlargement E[pq] : EX -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o o o | | | | | | | | | | | | | | | (dp) dq | | dp (dq) | | | | o<---------------->o<---------------->o | | | | | ^ | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 25-2. Difference Map D[pq] : EX -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / o \ \ | | / / ^ ^ \ \ | | o o / \ o o | | | | / \ | | | | | | / \ | | | | | |/ \| | | | | (dp)/ dq dp \(dq) | | | | /| |\ | | | | / | | \ | | | | / | | \ | | | o / o o \ o | | \ v \ dp dq / v / | | \ o<--------------------->o / | | \ \ / / | | \ \ / / | | \ \ / / | | \ o / | | \ / \ / | | o-------------------o o-------------------o | | | | | o---------------------------------------------------------------------o Figure 26-1. Differential or Tangent d[pq] : EX -> B |
o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o o o | | | | | | | | | | | | | | | | dp dq | | | | | o<------------------------------->o | | | | | | | | | | | | | | | | | o | | | | o o ^ o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ dp | dq / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | | | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 26-2. Remainder r[pq] : EX -> B |
JPEG Graphics
Series 1

|
| \(\text{Figure 22-a. Conjunction}~ pq : X \to \mathbb{B}\) |
Series 2

|
| \(\text{Figure 24-1. Proposition}~ pq : X \to \mathbb{B}\) |
Propositional Equation Reasoning Systems
Analysis of contingent propositions
 |
(26) |
 |
(27) |
| \(\text{Venn Diagram for}~ \texttt{(} p \texttt{~(} q \texttt{))~(} p \texttt{~(} r \texttt{))}\) |
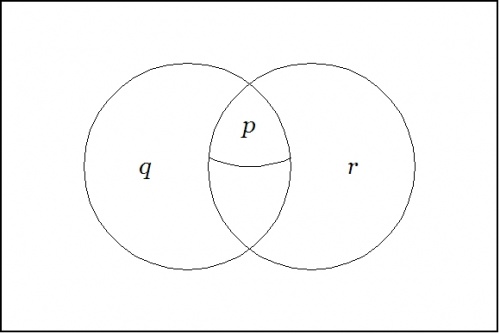 |
(28) |
| \(\text{Venn Diagram for}~ \texttt{(} p \texttt{~(} q ~ r \texttt{))}\) |
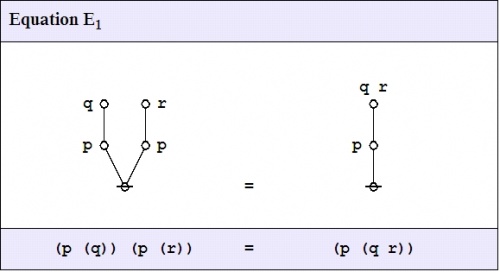 |
(29) |
Equation 1 : Proof 1
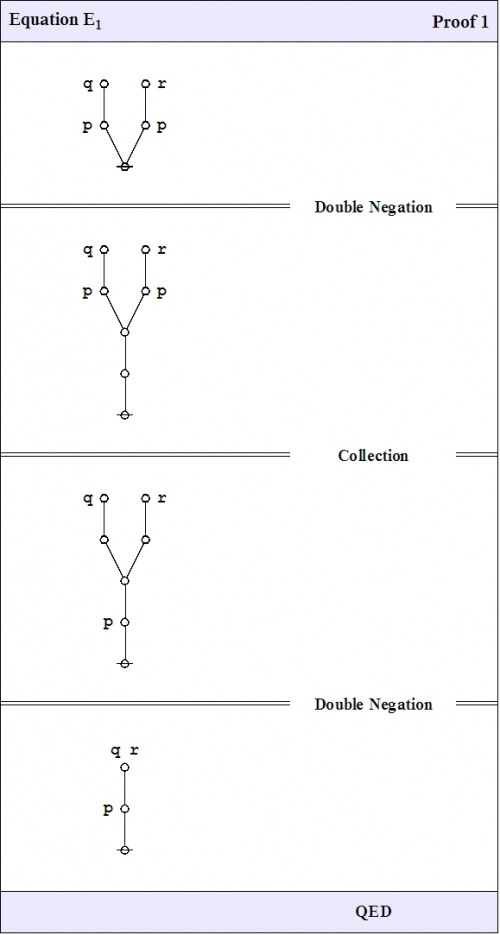
|
(30) |
Equation 1 : Proof 2
Single Image Version
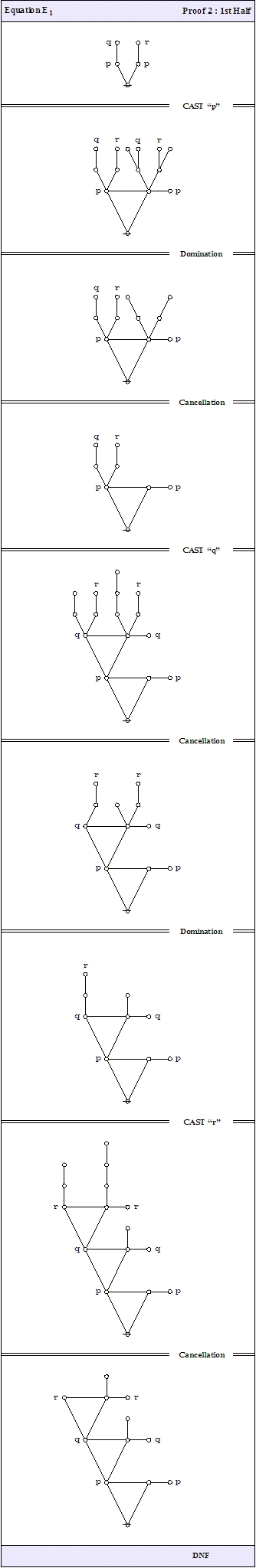
|
(31) |
Serial Image Version
|
(31) |
|
(33) |
Equation 1 : Proof 3
|
|
Variant 1
o-----------------------------------------------------------o | Equation E_1. Proof 3. | o-----------------------------------------------------------o | 1 | | q o o r q o r | | | | | | | p o o p p o | | \ / | | | o---------o | | \ / | | \ / | | \ / | | \ / | | o | | | | | | | | | | | | | | @ | | | o==================================< CAST "p" >=============o | 2 | | q r q r q r qr | | o o o o o o o o o | | | | | |/ |/ |/ | | o o o o o o | | \ / | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | p o---------------o---o p | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o==================================< Domination >===========o | 3 | | q r q r | | o o o o o o | | | | | / / / | | o o o o o o | | \ / | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | p o---------------o---o p | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 4 | | q r q r | | o o o | | | | | | | o o o | | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | p o---------------o---o p | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o==================================< Emptiness >============o | 5 | | q r q r | | o o o | | | | | | | o o o | | \ / | | | o-------o o | | \ / | | | \ / | | | \ / | | | o o | | | | | | | | | | | | | | p o---------------o---o p | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 6 | | q r q r | | o o o | | | | | | | o o o | | \ / | | | o-------o | | \ / | | \ / | | \ / | | o | | | | | | | | | | | p o---------------o---o p | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o==================================< CAST "q" >=============o | 7 | | o o | | r r | r | | | o o o o o o r | | | | | | | | | | o o o o o o | | \ / | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Domination >===========o | 8 | | o o | | r r | r | | | o o o o o o | | | | | | | | | | o o o o o o | | \ / | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 9 | | r r r | | o o o | | | | | | | o o o o o | | / | \ / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Domination >===========o | 10 | | r r | | o o | | | | | | o o o o | | / | \ | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Spike >================o | 11 | | r r | | o o | | | | | | o o | | / | | | o-------o o | | \ / | | | \ / | | | \ / | | | o o | | | | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 12 | | r r | | o o | | | | | | o o | | / | | | o-------o | | \ / | | \ / | | \ / | | o | | | | | | | | | | | q o---------------o---o q | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< CAST "r" >=============o | 13 | | o o | | | | | | o o o o | | | | | | | | o o o o | | / | / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | r o---------------o---o r | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | q o-------o---o q | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 14 | | o o | | / | | | o-------o o-------o | | \ / \ / | | \ / \ / | | \ / \ / | | o o | | | | | | | | | | | | | | r o---------------o---o r | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | q o-------o---o q | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Emptiness & Spike >====o | 15 | | o o 16 | | | | | | | | | | | | | | o o | | | | | | | | | | | | | | r o---------------o---o r | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | q o-------o---o q | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< Cancellation >=========o | 17 | | r o-------o---o r | | \ / | | \ / | | \ / | | q o-------o---o q | | \ / | | \ / | | \ / | | p o-------o---o p | | \ / | | \ / | | \ / | | @ | | | o==================================< QED >==================o |
(40) |
Variant 2
|
(40) |
Praeclarum Theorema : Proof by CAST
|
(23) |