Directory:Jon Awbrey/Papers/Dynamics And Logic

Note 1

I am going to excerpt some of my previous explorations on differential logic and dynamic systems and bring them to bear on the sorts of discrete dynamical themes that we find of interest in the NKS Forum. This adaptation draws on the "Cactus Rules", "Propositional Equation Reasoning Systems", and "Reductions Among Relations" threads, and will in time be applied to the "Differential Analytic Turing Automata" thread:

• CR. http://forum.wolframscience.com/showthread.php?threadid=256
• PERS. http://forum.wolframscience.com/showthread.php?threadid=297
• RAR. http://forum.wolframscience.com/showthread.php?threadid=400
• DATA. http://forum.wolframscience.com/showthread.php?threadid=228

One of the first things that you can do, once you have a moderately functional calculus for boolean functions or propositional logic, whatever you choose to call it, is to start thinking about, and even start computing, the differentials of these functions or propositions.

Let us start with a proposition of the form \(p ~\operatorname{and}~ q\) that is graphed as two labels attached to a root node:

o-------------------------------------------------o | | | p q | | @ | | | o-------------------------------------------------o | p and q | o-------------------------------------------------o

Written as a string, this is just the concatenation "\(p~q\)".

The proposition \(pq\!\) may be taken as a boolean function \(f(p, q)\!\) having the abstract type \(f : \mathbb{B} \times \mathbb{B} \to \mathbb{B},\) where \(\mathbb{B} = \{ 0, 1 \}\) is read in such a way that \(0\!\) means \(\operatorname{false}\) and \(1\!\) means \(\operatorname{true}.\)

In this style of graphical representation, the value \(\operatorname{true}\) looks like a blank label and the value \(\operatorname{false}\) looks like an edge.

o-------------------------------------------------o | | | | | @ | | | o-------------------------------------------------o | true | o-------------------------------------------------o

o-------------------------------------------------o | | | o | | | | | @ | | | o-------------------------------------------------o | false | o-------------------------------------------------o

Back to the proposition \(pq.\!\) Imagine yourself standing in a fixed cell of the corresponding venn diagram, say, the cell where the proposition \(pq\!\) is true, as shown here:

Now ask yourself: What is the value of the proposition \(pq\!\) at a distance of \(\operatorname{d}p\) and \(\operatorname{d}q\) from the cell \(pq\!\) where you are standing?

Don't think about it — just compute:

o-------------------------------------------------o | | | dp o o dq | | / \ / \ | | p o---@---o q | | | o-------------------------------------------------o | (p, dp) (q, dq) | o-------------------------------------------------o

To make future graphs easier to draw in ASCII, I will use devices like @=@=@ and o=o=o to identify several nodes into one, as in this next redrawing:

o-------------------------------------------------o | | | p dp q dq | | o---o o---o | | \ | | / | | \ | | / | | \| |/ | | @=@ | | | o-------------------------------------------------o | (p, dp) (q, dq) | o-------------------------------------------------o

However you draw it, these expressions follow because the expression \(p + \operatorname{d}p,\) where the plus sign indicates addition in \(\mathbb{B},\) that is, addition modulo 2, and thus corresponds to the exclusive disjunction operation in logic, parses to a graph of the following form:

o-------------------------------------------------o | | | p dp | | o---o | | \ / | | @ | | | o-------------------------------------------------o | (p, dp) | o-------------------------------------------------o

Next question: What is the difference between the value of the proposition \(pq\!\) "over there" and the value of the proposition \(pq\!\) where you are, all expressed in the form of a general formula, of course? Here is the appropriate formulation:

o-------------------------------------------------o | | | p dp q dq | | o---o o---o | | \ | | / | | \ | | / | | \| |/ p q | | o=o-----------o | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o-------------------------------------------------o | ((p, dp)(q, dq), p q) | o-------------------------------------------------o

There is one thing that I ought to mention at this point: Computed over \(\mathbb{B},\) plus and minus are identical operations. This will make the relation between the differential and the integral parts of the appropriate calculus slightly stranger than usual, but we will get into that later.

Last question, for now: What is the value of this expression from your current standpoint, that is, evaluated at the point where \(pq\!\) is true? Well, substituting \(1\!\) for \(p\!\) and \(1\!\) for \(q\!\) in the graph amounts to erasing the labels \(p\!\) and \(q\!,\) as shown here:

o-------------------------------------------------o | | | dp dq | | o---o o---o | | \ | | / | | \ | | / | | \| |/ | | o=o-----------o | | \ / | | \ / | | \ / | | \ / | | \ / | | \ / | | @ | | | o-------------------------------------------------o | (( , dp)( , dq), ) | o-------------------------------------------------o

And this is equivalent to the following graph:

o-------------------------------------------------o | | | dp dq | | o o | | \ / | | o | | | | | @ | | | o-------------------------------------------------o | ((dp) (dq)) | o-------------------------------------------------o

Note 2

We have just met with the fact that the differential of the and is the or of the differentials.

\(p ~\operatorname{and}~ q \quad \xrightarrow{~\operatorname{Diff}~} \quad \operatorname{d}p ~\operatorname{or}~ \operatorname{d}q\)

o-------------------------------------------------o | | | dp dq | | o o | | \ / | | o | | p q | | | @ --Diff--> @ | | | o-------------------------------------------------o | p q --Diff--> ((dp) (dq)) | o-------------------------------------------------o

It will be necessary to develop a more refined analysis of that statement directly, but that is roughly the nub of it.

If the form of the above statement reminds you of De Morgan's rule, it is no accident, as differentiation and negation turn out to be closely related operations. Indeed, one can find discussions of logical difference calculus in the Boole–De Morgan correspondence and Peirce also made use of differential operators in a logical context, but the exploration of these ideas has been hampered by a number of factors, not the least of which has been the lack of a syntax that was adequate to handle the complexity of expressions that evolve.

Let us run through the initial example again, this time attempting to interpret the formulas that develop at each stage along the way.

We begin with a proposition or a boolean function \(f(p, q) = pq.\!\)

A function like this has an abstract type and a concrete type. The abstract type is what we invoke when we write things like \(f : \mathbb{B} \times \mathbb{B} \to \mathbb{B}\) or \(f : \mathbb{B}^2 \to \mathbb{B}.\) The concrete type takes into account the qualitative dimensions or the "units" of the case, which can be explained as follows.

Let \(P\!\) be the set of values \(\{ \texttt{(} p \texttt{)},~ p \} ~=~ \{ \operatorname{not}~ p,~ p \} ~\cong~ \mathbb{B}.\)

Let \(Q\!\) be the set of values \(\{ \texttt{(} q \texttt{)},~ q \} ~=~ \{ \operatorname{not}~ q,~ q \} ~\cong~ \mathbb{B}.\)

Then interpret the usual propositions about \(p, q\!\) as functions of the concrete type \(f : P \times Q \to \mathbb{B}.\)

We are going to consider various operators on these functions. Here, an operator \(\operatorname{F}\) is a function that takes one function \(f\!\) into another function \(\operatorname{F}f.\)

The first couple of operators that we need to consider are logical analogues of the pair that play a founding role in the classical finite difference calculus, namely:

The difference operator \(\Delta,\!\) written here as \(\operatorname{D}.\)

The enlargement" operator \(\Epsilon,\!\) written here as \(\operatorname{E}.\)

These days, \(\operatorname{E}\) is more often called the shift operator.

In order to describe the universe in which these operators operate, it is necessary to enlarge the original universe of discourse. Starting from the initial space \(X = P \times Q,\) its (first order) differential extension \(\operatorname{E}X\) is constructed according to the following specifications:

\(\begin{array}{rcc} \operatorname{E}X & = & X \times \operatorname{d}X \end{array}\)

where:

\(\begin{array}{rcc} X & = & P \times Q \'"`UNIQ-MathJax1-QINU`"' Amazing! =='"`UNIQ--h-10--QINU`"'Note 11== We have been contemplating functions of the type \(f : X \to \mathbb{B}\) and studying the action of the operators \(\operatorname{E}\) and \(\operatorname{D}\) on this family. These functions, that we may identify for our present aims with propositions, inasmuch as they capture their abstract forms, are logical analogues of scalar potential fields. These are the sorts of fields that are so picturesquely presented in elementary calculus and physics textbooks by images of snow-covered hills and parties of skiers who trek down their slopes like least action heroes. The analogous scene in propositional logic presents us with forms more reminiscent of plateaunic idylls, being all plains at one of two levels, the mesas of verity and falsity, as it were, with nary a niche to inhabit between them, restricting our options for a sporting gradient of downhill dynamics to just one of two: standing still on level ground or falling off a bluff. We are still working well within the logical analogue of the classical finite difference calculus, taking in the novelties that the logical transmutation of familiar elements is able to bring to light. Soon we will take up several different notions of approximation relationships that may be seen to organize the space of propositions, and these will allow us to define several different forms of differential analysis applying to propositions. In time we will find reason to consider more general types of maps, having concrete types of the form \(X_1 \times \ldots \times X_k \to Y_1 \times \ldots \times Y_n\) and abstract types \(\mathbb{B}^k \to \mathbb{B}^n.\) We will think of these mappings as transforming universes of discourse into themselves or into others, in short, as transformations of discourse. Before we continue with this intinerary, however, I would like to highlight another sort of differential aspect that concerns the boundary operator or the marked connective that serves as one of the two basic connectives in the cactus language for ZOL. For example, consider the proposition \(f\!\) of concrete type \(f : P \times Q \times R \to \mathbb{B}\) and abstract type \(f : \mathbb{B}^3 \to \mathbb{B}\) that is written \(\texttt{(} p, q, r \texttt{)}\) in cactus syntax. Taken as an assertion in what Peirce called the existential interpretation, the proposition \(\texttt{(} p, q, r \texttt{)}\) says that just one of \(p, q, r\!\) is false. It is instructive to consider this assertion in relation to the logical conjunction \(pqr\!\) of the same propositions. A venn diagram of \(\texttt{(} p, q, r \texttt{)}\) looks like this: In relation to the center cell indicated by the conjunction \(pqr,\!\) the region indicated by \(\texttt{(} p, q, r \texttt{)}\) is comprised of the adjacent or bordering cells. Thus they are the cells that are just across the boundary of the center cell, reached as if by way of Leibniz's minimal changes from the point of origin, in this case, \(pqr.\!\) More generally speaking, in a \(k\!\)-dimensional universe of discourse that is based on the alphabet of features \(\mathcal{X} = \{ x_1, \ldots, x_k \},\) the same form of boundary relationship is manifested for any cell of origin that one chooses to indicate. One way to indicate a cell is by forming a logical conjunction of positive and negative basis features, that is, by constructing an expression of the form \(e_1 \cdot \ldots \cdot e_k,\) where \(e_j = x_j ~\text{or}~ e_j = \texttt{(} x_j \texttt{)},\) for \(j = 1 ~\text{to}~ k.\) The proposition \(\texttt{(} e_1, \ldots, e_k \texttt{)}\) indicates the disjunctive region consisting of the cells that are just next door to \(e_1 \cdot \ldots \cdot e_k.\) Note 12 Consider what effects that might conceivably have practical bearings you conceive the objects of your conception to have. Then, your conception of those effects is the whole of your conception of the object. — Charles Sanders Peirce, "Issues of Pragmaticism", (CP 5.438) One other subject that it would be opportune to mention at this point, while we have an object example of a mathematical group fresh in mind, is the relationship between the pragmatic maxim and what are commonly known in mathematics as representation principles. As it turns out, with regard to its formal characteristics, the pragmatic maxim unites the aspects of a representation principle with the attributes of what would ordinarily be known as a closure principle. We will consider the form of closure that is invoked by the pragmatic maxim on another occasion, focusing here and now on the topic of group representations. Let us return to the example of the four-group \(V_4.\!\) We encountered this group in one of its concrete representations, namely, as a transformation group that acts on a set of objects, in this case a set of sixteen functions or propositions. Forgetting about the set of objects that the group transforms among themselves, we may take the abstract view of the group's operational structure, for example, in the form of the group operation table copied here: \(\cdot\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{e}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{f}\) \(\operatorname{f}\) \(\operatorname{e}\) \(\operatorname{h}\) \(\operatorname{g}\) \(\operatorname{g}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{h}\) \(\operatorname{h}\) \(\operatorname{g}\) \(\operatorname{f}\) \(\operatorname{e}\) This table is abstractly the same as, or isomorphic to, the versions with the \(\operatorname{E}_{ij}\) operators and the \(\operatorname{T}_{ij}\) transformations that we took up earlier. That is to say, the story is the same, only the names have been changed. An abstract group can have a variety of significantly and superficially different representations. But even after we have long forgotten the details of any particular representation there is a type of concrete representations, called regular representations, that are always readily available, as they can be generated from the mere data of the abstract operation table itself. To see how a regular representation is constructed from the abstract operation table, select a group element from the top margin of the Table, and "consider its effects" on each of the group elements as they are listed along the left margin. We may record these effects as Peirce usually did, as a logical aggregate of elementary dyadic relatives, that is, as a logical disjunction or boolean sum whose terms represent the ordered pairs of \(\operatorname{input} : \operatorname{output}\) transactions that are produced by each group element in turn. This forms one of the two possible regular representations of the group, in this case the one that is called the post-regular representation or the right regular representation. It has long been conventional to organize the terms of this logical aggregate in the form of a matrix: Reading "\(+\!\)" as a logical disjunction: \(\begin{matrix} \operatorname{G} & = & \operatorname{e} & + & \operatorname{f} & + & \operatorname{g} & + & \operatorname{h} \end{matrix}\) And so, by expanding effects, we get: \(\begin{matrix} \operatorname{G} & = & \operatorname{e}:\operatorname{e} & + & \operatorname{f}:\operatorname{f} & + & \operatorname{g}:\operatorname{g} & + & \operatorname{h}:\operatorname{h} \'"`UNIQ-MathJax2-QINU`"' is the relate, \(j\!\) is the correlate, and in our current example \(i\!:\!j,\) or more exactly, \(m_{ij} = 1,\!\) is taken to say that \(i\!\) is a marker for \(j.\!\) This is the mode of reading that we call "multiplying on the left". In the algebraic, permutational, or transformational contexts of application, however, Peirce converts to the alternative mode of reading, although still calling \(i\!\) the relate and \(j\!\) the correlate, the elementary relative \(i\!:\!j\) now means that \(i\!\) gets changed into \(j.\!\) In this scheme of reading, the transformation \(a\!:\!b + b\!:\!c + c\!:\!a\) is a permutation of the aggregate \(\mathbf{1} = a + b + c,\) or what we would now call the set \(\{ a, b, c \},\!\) in particular, it is the permutation that is otherwise notated as follows: \(\begin{Bmatrix} a & b & c \\ b & c & a \end{Bmatrix}\) This is consistent with the convention that Peirce uses in the paper "On a Class of Multiple Algebras" (CP 3.324–327). Note 16 We've been exploring the applications of a certain technique for clarifying abstruse concepts, a rough-cut version of the pragmatic maxim that I've been accustomed to refer to as the operationalization of ideas. The basic idea is to replace the question of What it is, which modest people comprehend is far beyond their powers to answer definitively any time soon, with the question of What it does, which most people know at least a modicum about. In the case of regular representations of groups we found a non-plussing surplus of answers to sort our way through. So let us track back one more time to see if we can learn any lessons that might carry over to more realistic cases. Here is is the operation table of \(V_4\!\) once again: \(\text{Klein Four-Group}~ V_4\) \(\cdot\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{e}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{f}\) \(\operatorname{f}\) \(\operatorname{e}\) \(\operatorname{h}\) \(\operatorname{g}\) \(\operatorname{g}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{h}\) \(\operatorname{h}\) \(\operatorname{g}\) \(\operatorname{f}\) \(\operatorname{e}\) A group operation table is really just a device for recording a certain 3-adic relation, to be specific, the set of triples of the form \((x, y, z)\!\) satisfying the equation \(x \cdot y = z.\) In the case of \(V_4 = (G, \cdot),\) where \(G\!\) is the underlying set \(\{ \operatorname{e}, \operatorname{f}, \operatorname{g}, \operatorname{h} \},\) we have the 3-adic relation \(L(V_4) \subseteq G \times G \times G\) whose triples are listed below: \(\begin{matrix} (\operatorname{e}, \operatorname{e}, \operatorname{e}) & (\operatorname{e}, \operatorname{f}, \operatorname{f}) & (\operatorname{e}, \operatorname{g}, \operatorname{g}) & (\operatorname{e}, \operatorname{h}, \operatorname{h}) \\[6pt] (\operatorname{f}, \operatorname{e}, \operatorname{f}) & (\operatorname{f}, \operatorname{f}, \operatorname{e}) & (\operatorname{f}, \operatorname{g}, \operatorname{h}) & (\operatorname{f}, \operatorname{h}, \operatorname{g}) \\[6pt] (\operatorname{g}, \operatorname{e}, \operatorname{g}) & (\operatorname{g}, \operatorname{f}, \operatorname{h}) & (\operatorname{g}, \operatorname{g}, \operatorname{e}) & (\operatorname{g}, \operatorname{h}, \operatorname{f}) \\[6pt] (\operatorname{h}, \operatorname{e}, \operatorname{h}) & (\operatorname{h}, \operatorname{f}, \operatorname{g}) & (\operatorname{h}, \operatorname{g}, \operatorname{f}) & (\operatorname{h}, \operatorname{h}, \operatorname{e}) \end{matrix}\) It is part of the definition of a group that the 3-adic relation \(L \subseteq G^3\) is actually a function \(L : G \times G \to G.\) It is from this functional perspective that we can see an easy way to derive the two regular representations. Since we have a function of the type \(L : G \times G \to G,\) we can define a couple of substitution operators: 1. \(\operatorname{Sub}(x, (\underline{~~}, y))\) puts any specified \(x\!\) into the empty slot of the rheme \((\underline{~~}, y),\) with the effect of producing the saturated rheme \((x, y)\!\) that evaluates to \(xy.\!\) 2. \(\operatorname{Sub}(x, (y, \underline{~~}))\) puts any specified \(x\!\) into the empty slot of the rheme \((y, \underline{~~}),\) with the effect of producing the saturated rheme \((y, x)\!\) that evaluates to \(yx.\!\) In (1) we consider the effects of each \(x\!\) in its practical bearing on contexts of the form \((\underline{~~}, y),\) as \(y\!\) ranges over \(G,\!\) and the effects are such that \(x\!\) takes \((\underline{~~}, y)\) into \(xy,\!\) for \(y\!\) in \(G,\!\) all of which is notated as \(x = \{ (y : xy) ~|~ y \in G \}.\) The pairs \((y : xy)\!\) can be found by picking an \(x\!\) from the left margin of the group operation table and considering its effects on each \(y\!\) in turn as these run across the top margin. This aspect of pragmatic definition we recognize as the regular ante-representation: \(\begin{matrix} \operatorname{e} & = & \operatorname{e}\!:\!\operatorname{e} & + & \operatorname{f}\!:\!\operatorname{f} & + & \operatorname{g}\!:\!\operatorname{g} & + & \operatorname{h}\!:\!\operatorname{h} \\[4pt] \operatorname{f} & = & \operatorname{e}\!:\!\operatorname{f} & + & \operatorname{f}\!:\!\operatorname{e} & + & \operatorname{g}\!:\!\operatorname{h} & + & \operatorname{h}\!:\!\operatorname{g} \\[4pt] \operatorname{g} & = & \operatorname{e}\!:\!\operatorname{g} & + & \operatorname{f}\!:\!\operatorname{h} & + & \operatorname{g}\!:\!\operatorname{e} & + & \operatorname{h}\!:\!\operatorname{f} \\[4pt] \operatorname{h} & = & \operatorname{e}\!:\!\operatorname{h} & + & \operatorname{f}\!:\!\operatorname{g} & + & \operatorname{g}\!:\!\operatorname{f} & + & \operatorname{h}\!:\!\operatorname{e} \end{matrix}\) In (2) we consider the effects of each \(x\!\) in its practical bearing on contexts of the form \((y, \underline{~~}),\) as \(y\!\) ranges over \(G,\!\) and the effects are such that \(x\!\) takes \((y, \underline{~~})\) into \(yx,\!\) for \(y\!\) in \(G,\!\) all of which is notated as \(x = \{ (y : yx) ~|~ y \in G \}.\) The pairs \((y : yx)\!\) can be found by picking an \(x\!\) from the top margin of the group operation table and considering its effects on each \(y\!\) in turn as these run down the left margin. This aspect of pragmatic definition we recognize as the regular post-representation: \(\begin{matrix} \operatorname{e} & = & \operatorname{e}\!:\!\operatorname{e} & + & \operatorname{f}\!:\!\operatorname{f} & + & \operatorname{g}\!:\!\operatorname{g} & + & \operatorname{h}\!:\!\operatorname{h} \\[4pt] \operatorname{f} & = & \operatorname{e}\!:\!\operatorname{f} & + & \operatorname{f}\!:\!\operatorname{e} & + & \operatorname{g}\!:\!\operatorname{h} & + & \operatorname{h}\!:\!\operatorname{g} \\[4pt] \operatorname{g} & = & \operatorname{e}\!:\!\operatorname{g} & + & \operatorname{f}\!:\!\operatorname{h} & + & \operatorname{g}\!:\!\operatorname{e} & + & \operatorname{h}\!:\!\operatorname{f} \\[4pt] \operatorname{h} & = & \operatorname{e}\!:\!\operatorname{h} & + & \operatorname{f}\!:\!\operatorname{g} & + & \operatorname{g}\!:\!\operatorname{f} & + & \operatorname{h}\!:\!\operatorname{e} \end{matrix}\) If the ante-rep looks the same as the post-rep, now that I'm writing them in the same dialect, that is because \(V_4\!\) is abelian (commutative), and so the two representations have the very same effects on each point of their bearing. Note 17 So long as we're in the neighborhood, we might as well take in some more of the sights, for instance, the smallest example of a non-abelian (non-commutative) group. This is a group of six elements, say, \(G = \{ \operatorname{e}, \operatorname{f}, \operatorname{g}, \operatorname{h}, \operatorname{i}, \operatorname{j} \},\!\) with no relation to any other employment of these six symbols being implied, of course, and it can be most easily represented as the permutation group on a set of three letters, say, \(X = \{ a, b, c \},\!\) usually notated as \(G = \operatorname{Sym}(X)\) or more abstractly and briefly, as \(\operatorname{Sym}(3)\) or \(S_3.\!\) The next Table shows the intended correspondence between abstract group elements and the permutation or substitution operations in \(\operatorname{Sym}(X).\) \(\text{Permutation Substitutions in}~ \operatorname{Sym} \{ a, b, c \}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{i}\) \(\operatorname{j}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] a & b & c \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] c & a & b \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] b & c & a \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] a & c & b \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] c & b & a \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] b & a & c \end{matrix}\) Here is the operation table for \(S_3,\!\) given in abstract fashion: Symmetric Group S_3 o-------------------------------------------------o | | | ^ | | e / \ e | | / \ | | / e \ | | f / \ / \ f | | / \ / \ | | / f \ f \ | | g / \ / \ / \ g | | / \ / \ / \ | | / g \ g \ g \ | | h / \ / \ / \ / \ h | | / \ / \ / \ / \ | | / h \ e \ e \ h \ | | i / \ / \ / \ / \ / \ i | | / \ / \ / \ / \ / \ | | / i \ i \ f \ j \ i \ | | j / \ / \ / \ / \ / \ / \ j | | / \ / \ / \ / \ / \ / \ | | ( j \ j \ j \ i \ h \ j ) | | \ / \ / \ / \ / \ / \ / | | \ / \ / \ / \ / \ / \ / | | \ h \ h \ e \ j \ i / | | \ / \ / \ / \ / \ / | | \ / \ / \ / \ / \ / | | \ i \ g \ f \ h / | | \ / \ / \ / \ / | | \ / \ / \ / \ / | | \ f \ e \ g / | | \ / \ / \ / | | \ / \ / \ / | | \ g \ f / | | \ / \ / | | \ / \ / | | \ e / | | \ / | | \ / | | v | | | o-------------------------------------------------o By the way, we will meet with the symmetric group \(S_3\!\) again when we return to take up the study of Peirce's early paper "On a Class of Multiple Algebras" (CP 3.324–327), and also his late unpublished work "The Simplest Mathematics" (1902) (CP 4.227–323), with particular reference to the section that treats of "Trichotomic Mathematics" (CP 4.307–323). Note 18 By way of collecting a short-term pay-off for all the work that we did on the regular representations of the Klein 4-group \(V_4,\!\) let us write out as quickly as possible in relative form a minimal budget of representations for the symmetric group on three letters, \(\operatorname{Sym}(3).\) After doing the usual bit of compare and contrast among the various representations, we will have enough concrete material beneath our abstract belts to tackle a few of the presently obscured details of Peirce's early "Algebra + Logic" papers. Writing the permutations or substitutions of \(\operatorname{Sym} \{ a, b, c \}\) in relative form generates what is generally thought of as a natural representation of \(S_3.\!\) \(\begin{matrix} \operatorname{e} & = & a\!:\!a & + & b\!:\!b & + & c\!:\!c \\[4pt] \operatorname{f} & = & a\!:\!c & + & b\!:\!a & + & c\!:\!b \\[4pt] \operatorname{g} & = & a\!:\!b & + & b\!:\!c & + & c\!:\!a \\[4pt] \operatorname{h} & = & a\!:\!a & + & b\!:\!c & + & c\!:\!b \\[4pt] \operatorname{i} & = & a\!:\!c & + & b\!:\!b & + & c\!:\!a \\[4pt] \operatorname{j} & = & a\!:\!b & + & b\!:\!a & + & c\!:\!c \end{matrix}\) I have without stopping to think about it written out this natural representation of \(S_3\!\) in the style that comes most naturally to me, to wit, the "right" way, whereby an ordered pair configured as \(x\!:\!y\) constitutes the turning of \(x\!\) into \(y.\!\) It is possible that the next time we check in with CSP we will have to adjust our sense of direction, but that will be an easy enough bridge to cross when we come to it. Note 19 To construct the regular representations of \(S_3,\!\) we begin with the data of its operation table: Symmetric Group S_3 o-------------------------------------------------o | | | ^ | | e / \ e | | / \ | | / e \ | | f / \ / \ f | | / \ / \ | | / f \ f \ | | g / \ / \ / \ g | | / \ / \ / \ | | / g \ g \ g \ | | h / \ / \ / \ / \ h | | / \ / \ / \ / \ | | / h \ e \ e \ h \ | | i / \ / \ / \ / \ / \ i | | / \ / \ / \ / \ / \ | | / i \ i \ f \ j \ i \ | | j / \ / \ / \ / \ / \ / \ j | | / \ / \ / \ / \ / \ / \ | | ( j \ j \ j \ i \ h \ j ) | | \ / \ / \ / \ / \ / \ / | | \ / \ / \ / \ / \ / \ / | | \ h \ h \ e \ j \ i / | | \ / \ / \ / \ / \ / | | \ / \ / \ / \ / \ / | | \ i \ g \ f \ h / | | \ / \ / \ / \ / | | \ / \ / \ / \ / | | \ f \ e \ g / | | \ / \ / \ / | | \ / \ / \ / | | \ g \ f / | | \ / \ / | | \ / \ / | | \ e / | | \ / | | \ / | | v | | | o-------------------------------------------------o Just by way of staying clear about what we are doing, let's return to the recipe that we worked out before: It is part of the definition of a group that the 3-adic relation \(L \subseteq G^3\) is actually a function \(L : G \times G \to G.\) It is from this functional perspective that we can see an easy way to derive the two regular representations. Since we have a function of the type \(L : G \times G \to G,\) we can define a couple of substitution operators: 1. \(\operatorname{Sub}(x, (\underline{~~}, y))\) puts any specified \(x\!\) into the empty slot of the rheme \((\underline{~~}, y),\) with the effect of producing the saturated rheme \((x, y)\!\) that evaluates to \(xy.\!\) 2. \(\operatorname{Sub}(x, (y, \underline{~~}))\) puts any specified \(x\!\) into the empty slot of the rheme \((y, \underline{~~}),\) with the effect of producing the saturated rheme \((y, x)\!\) that evaluates to \(yx.\!\) In (1) we consider the effects of each \(x\!\) in its practical bearing on contexts of the form \((\underline{~~}, y),\) as \(y\!\) ranges over \(G,\!\) and the effects are such that \(x\!\) takes \((\underline{~~}, y)\) into \(xy,\!\) for \(y\!\) in \(G,\!\) all of which is notated as \(x = \{ (y : xy) ~|~ y \in G \}.\) The pairs \((y : xy)\!\) can be found by picking an \(x\!\) from the left margin of the group operation table and considering its effects on each \(y\!\) in turn as these run along the right margin. This produces the regular ante-representation of \(S_3,\!\) like so: \(\begin{array}{*{13}{c}} \operatorname{e} & = & \operatorname{e}\!:\!\operatorname{e} & + & \operatorname{f}\!:\!\operatorname{f} & + & \operatorname{g}\!:\!\operatorname{g} & + & \operatorname{h}\!:\!\operatorname{h} & + & \operatorname{i}\!:\!\operatorname{i} & + & \operatorname{j}\!:\!\operatorname{j} \\[4pt] \operatorname{f} & = & \operatorname{e}\!:\!\operatorname{f} & + & \operatorname{f}\!:\!\operatorname{g} & + & \operatorname{g}\!:\!\operatorname{e} & + & \operatorname{h}\!:\!\operatorname{j} & + & \operatorname{i}\!:\!\operatorname{h} & + & \operatorname{j}\!:\!\operatorname{i} \\[4pt] \operatorname{g} & = & \operatorname{e}\!:\!\operatorname{g} & + & \operatorname{f}\!:\!\operatorname{e} & + & \operatorname{g}\!:\!\operatorname{f} & + & \operatorname{h}\!:\!\operatorname{i} & + & \operatorname{i}\!:\!\operatorname{j} & + & \operatorname{j}\!:\!\operatorname{h} \\[4pt] \operatorname{h} & = & \operatorname{e}\!:\!\operatorname{h} & + & \operatorname{f}\!:\!\operatorname{i} & + & \operatorname{g}\!:\!\operatorname{j} & + & \operatorname{h}\!:\!\operatorname{e} & + & \operatorname{i}\!:\!\operatorname{f} & + & \operatorname{j}\!:\!\operatorname{g} \\[4pt] \operatorname{i} & = & \operatorname{e}\!:\!\operatorname{i} & + & \operatorname{f}\!:\!\operatorname{j} & + & \operatorname{g}\!:\!\operatorname{h} & + & \operatorname{h}\!:\!\operatorname{g} & + & \operatorname{i}\!:\!\operatorname{e} & + & \operatorname{j}\!:\!\operatorname{f} \\[4pt] \operatorname{j} & = & \operatorname{e}\!:\!\operatorname{j} & + & \operatorname{f}\!:\!\operatorname{h} & + & \operatorname{g}\!:\!\operatorname{i} & + & \operatorname{h}\!:\!\operatorname{f} & + & \operatorname{i}\!:\!\operatorname{g} & + & \operatorname{j}\!:\!\operatorname{e} \end{array}\) In (2) we consider the effects of each \(x\!\) in its practical bearing on contexts of the form \((y, \underline{~~}),\) as \(y\!\) ranges over \(G,\!\) and the effects are such that \(x\!\) takes \((y, \underline{~~})\) into \(yx,\!\) for \(y\!\) in \(G,\!\) all of which is notated as \(x = \{ (y : yx) ~|~ y \in G \}.\) The pairs \((y : yx)\!\) can be found by picking an \(x\!\) on the right margin of the group operation table and considering its effects on each \(y\!\) in turn as these run along the left margin. This produces the regular post-representation of \(S_3,\!\) like so: \(\begin{array}{*{13}{c}} \operatorname{e} & = & \operatorname{e}\!:\!\operatorname{e} & + & \operatorname{f}\!:\!\operatorname{f} & + & \operatorname{g}\!:\!\operatorname{g} & + & \operatorname{h}\!:\!\operatorname{h} & + & \operatorname{i}\!:\!\operatorname{i} & + & \operatorname{j}\!:\!\operatorname{j} \\[4pt] \operatorname{f} & = & \operatorname{e}\!:\!\operatorname{f} & + & \operatorname{f}\!:\!\operatorname{g} & + & \operatorname{g}\!:\!\operatorname{e} & + & \operatorname{h}\!:\!\operatorname{i} & + & \operatorname{i}\!:\!\operatorname{j} & + & \operatorname{j}\!:\!\operatorname{h} \\[4pt] \operatorname{g} & = & \operatorname{e}\!:\!\operatorname{g} & + & \operatorname{f}\!:\!\operatorname{e} & + & \operatorname{g}\!:\!\operatorname{f} & + & \operatorname{h}\!:\!\operatorname{j} & + & \operatorname{i}\!:\!\operatorname{h} & + & \operatorname{j}\!:\!\operatorname{i} \\[4pt] \operatorname{h} & = & \operatorname{e}\!:\!\operatorname{h} & + & \operatorname{f}\!:\!\operatorname{j} & + & \operatorname{g}\!:\!\operatorname{i} & + & \operatorname{h}\!:\!\operatorname{e} & + & \operatorname{i}\!:\!\operatorname{g} & + & \operatorname{j}\!:\!\operatorname{f} \\[4pt] \operatorname{i} & = & \operatorname{e}\!:\!\operatorname{i} & + & \operatorname{f}\!:\!\operatorname{h} & + & \operatorname{g}\!:\!\operatorname{j} & + & \operatorname{h}\!:\!\operatorname{f} & + & \operatorname{i}\!:\!\operatorname{e} & + & \operatorname{j}\!:\!\operatorname{g} \\[4pt] \operatorname{j} & = & \operatorname{e}\!:\!\operatorname{j} & + & \operatorname{f}\!:\!\operatorname{i} & + & \operatorname{g}\!:\!\operatorname{h} & + & \operatorname{h}\!:\!\operatorname{g} & + & \operatorname{i}\!:\!\operatorname{f} & + & \operatorname{j}\!:\!\operatorname{e} \end{array}\) If the ante-rep looks different from the post-rep, it is just as it should be, as \(S_3\!\) is non-abelian (non-commutative), and so the two representations differ in the details of their practical effects, though, of course, being representations of the same abstract group, they must be isomorphic. Note 20   the way of heaven and earth is to be long continued in their operation without stopping   — i ching, hexagram 32 The Reader may be wondering what happened to the announced subject of Dynamics And Logic. What happened was a bit like this: We made the observation that the shift operators \(\{ \operatorname{E}_{ij} \}\) form a transformation group that acts on the set of propositions of the form \(f : \mathbb{B} \times \mathbb{B} \to \mathbb{B}.\) Group theory is a very attractive subject, but it did not draw us so far from our intended course as one might initially think. For one thing, groups, especially the groups that are named after the Norwegian mathematician Marius Sophus Lie (1842–1899), have turned out to be of critical utility in the solution of differential equations. For another thing, group operations provide us with an ample supply of triadic relations that have been extremely well-studied over the years, and thus they give us no small measure of useful guidance in the study of sign relations, another brand of 3-adic relations that have significance for logical studies, and in our acquaintance with which we have barely begun to break the ice. Finally, I couldn't resist taking up the links between group representations, amounting to the very archetypes of logical models, and the pragmatic maxim. Note 21 We've seen a couple of groups, \(V_4\!\) and \(S_3,\!\) represented in various ways, and we've seen their representations presented in a variety of different manners. Let us look at one other stylistic variant for presenting a representation that is frequently seen, the so-called matrix representation of a group. Recalling the manner of our acquaintance with the symmetric group \(S_3,\!\) we began with the bigraph (bipartite graph) picture of its natural representation as the set of all permutations or substitutions on the set \(X = \{ a, b, c \}.\!\) \(\text{Permutation Substitutions in}~ \operatorname{Sym} \{ a, b, c \}\) \(\operatorname{e}\) \(\operatorname{f}\) \(\operatorname{g}\) \(\operatorname{h}\) \(\operatorname{i}\) \(\operatorname{j}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] a & b & c \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] c & a & b \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] b & c & a \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] a & c & b \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] c & b & a \end{matrix}\) \(\begin{matrix} a & b & c \\[3pt] \downarrow & \downarrow & \downarrow \\[6pt] b & a & c \end{matrix}\) These permutations were then converted to relative form as logical sums of elementary relatives: \(\begin{matrix} \operatorname{e} & = & a\!:\!a & + & b\!:\!b & + & c\!:\!c \\[4pt] \operatorname{f} & = & a\!:\!c & + & b\!:\!a & + & c\!:\!b \\[4pt] \operatorname{g} & = & a\!:\!b & + & b\!:\!c & + & c\!:\!a \\[4pt] \operatorname{h} & = & a\!:\!a & + & b\!:\!c & + & c\!:\!b \\[4pt] \operatorname{i} & = & a\!:\!c & + & b\!:\!b & + & c\!:\!a \\[4pt] \operatorname{j} & = & a\!:\!b & + & b\!:\!a & + & c\!:\!c \end{matrix}\) From this relational representation of Sym {a, b, c} ~=~ S_3, one easily derives a "linear representation", regarding each permutation as a linear transformation that maps the elements of a suitable vector space into each other, and representing each of these linear transformations by means of a matrix, resulting in the following set of matrices for the group: Table 21. Matrix Representations of the Permutations in S_3 o---------o---------o---------o---------o---------o---------o | | | | | | | | e | f | g | h | i | j | | | | | | | | o=========o=========o=========o=========o=========o=========o | | | | | | | | 1 0 0 | 0 0 1 | 0 1 0 | 1 0 0 | 0 0 1 | 0 1 0 | | 0 1 0 | 1 0 0 | 0 0 1 | 0 0 1 | 0 1 0 | 1 0 0 | | 0 0 1 | 0 1 0 | 1 0 0 | 0 1 0 | 1 0 0 | 0 0 1 | | | | | | | | o---------o---------o---------o---------o---------o---------o The key to the mysteries of these matrices is revealed by observing that their coefficient entries are arrayed and overlayed on a place mat that's marked like so: o-----o-----o-----o | a:a | a:b | a:c | o-----o-----o-----o | b:a | b:b | b:c | o-----o-----o-----o | c:a | c:b | c:c | o-----o-----o-----o Note 22 It would be good to summarize, in rough but intuitive terms, the outlook on differential logic that we have reached so far. We've been considering a class of operators on universes of discourse, each of which takes us from considering one universe of discourse, X%, to considering a larger universe of discourse, EX%. Each of these operators, in broad terms having the form W : X% -> EX%, acts on each proposition f : X -> B of the source universe X% to produce a proposition Wf : EX -> B of the target universe EX%. The two main operators that we have worked with up to this point are the enlargement or shift operator E : X% -> EX% and the difference operator D : X% -> EX%. E and D take a proposition in X%, that is, a proposition f : X -> B that is said to be "about" the subject matter of X, and produce the extended propositions Ef, Df : EX -> B, which may be interpreted as being about specified collections of changes that might occur in X. Here we have need of visual representations, some array of concrete pictures to anchor our more earthy intuitions and to help us keep our wits about us before we try to climb any higher into the ever more rarefied air of abstractions. One good picture comes to us by way of the "field" concept. Given a space X, a "field" of a specified type Y over X is formed by assigning to each point of X an object of type Y. If that sounds like the same thing as a function from X to the space of things of type Y -- it is -- but it does seem helpful to vary the mental images and to take advantage of the figures of speech that spring to mind under the emblem of this field idea. In the field picture, a proposition f : X -> B becomes a "scalar" field, that is, a field of values in B, or a "field of model indications" (FOMI). 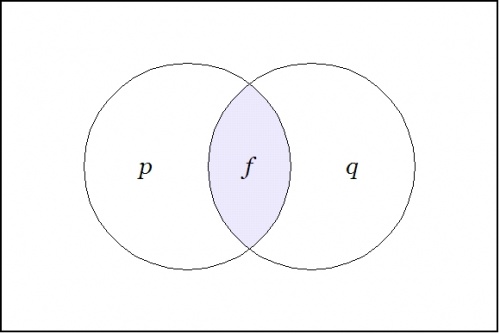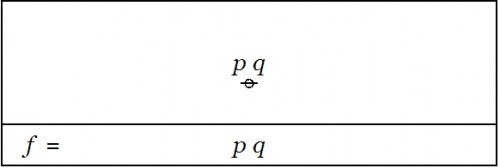Let us take a moment to view an old proposition in this new light, for example, the conjunction pq : X -> B that is depicted in Figure 22-a. o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / o \ | | / /%\ \ | | / /%%%\ \ | | o o%%%%%o o | | | |%%%%%| | | | | P |%%%%%| Q | | | | |%%%%%| | | | o o%%%%%o o | | \ \%%%/ / | | \ \%/ / | | \ o / | | \ / \ / | | o-------------o o-------------o | | | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o Figure 22-a. Conjunction pq : X -> B Each of the operators E, D : X% -> EX% takes us from considering propositions f : X -> B, here viewed as "scalar fields" over X, to considering the corresponding "differential fields" over X, analogous to what are usually called "vector fields" over X. The structure of these differential fields can be described this way. To each point of X there is attached an object of the following type: a proposition about changes in X, that is, a proposition g : dX -> B. In this frame, if X% is the universe that is generated by the set of coordinate propositions {p, q}, then dX% is the differential universe that is generated by the set of differential propositions {dp, dq}. These differential propositions may be interpreted as indicating "change in p" and "change in q", respectively. A differential operator W, of the first order sort that we have been considering, takes a proposition f : X -> B and gives back a differential proposition Wf: EX -> B. In the field view, we see the proposition f : X -> B as a scalar field and we see the differential proposition Wf: EX -> B as a vector field, specifically, a field of propositions about contemplated changes in X. The field of changes produced by E on pq is shown in Figure 22-b. o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / P o Q \ | | / /%\ \ | | / /%%%\ \ | | o o.->-.o o | | | p(q)(dp)dq |%\%/%| (p)q dp(dq) | | | | o---------------|->o<-|---------------o | | | | |%%^%%| | | | o o%%|%%o o | | \ \%|%/ / | | \ \|/ / | | \ o / | | \ /|\ / | | o-------------o | o-------------o | | | | | | | | | | | o | | (p)(q) dp dq | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o | | | Ef = p q (dp)(dq) | | | | + p (q) (dp) dq | | | | + (p) q dp (dq) | | | | + (p)(q) dp dq | | | o-------------------------------------------------o Figure 22-b. Enlargement E[pq] : EX -> B The differential field E[pq] specifies the changes that need to be made from each point of X in order to reach one of the models of the proposition pq, that is, in order to satisfy the proposition pq. The field of changes produced by D on pq is shown in Figure 22-c. o-------------------------------------------------o | | | | | o-------------o o-------------o | | / \ / \ | | / P o Q \ | | / /%\ \ | | / /%%%\ \ | | o o%%%%%o o | | | (dp)dq |%%%%%| dp(dq) | | | | o<--------------|->o<-|-------------->o | | | | |%%^%%| | | | o o%%|%%o o | | \ \%|%/ / | | \ \|/ / | | \ o / | | \ /|\ / | | o-------------o | o-------------o | | | | | | | | v | | o | | dp dq | | | o-------------------------------------------------o | f = p q | o-------------------------------------------------o | | | Df = p q ((dp)(dq)) | | | | + p (q) (dp) dq | | | | + (p) q dp (dq) | | | | + (p)(q) dp dq | | | o-------------------------------------------------o Figure 22-c. Difference D[pq] : EX -> B The differential field D[pq] specifies the changes that need to be made from each point of X in order to feel a change in the felt value of the field pq. Note 23 I want to continue developing the basic tools of differential logic, which arose out of many years of thinking about the connections between dynamics and logic -- those there are and those there ought to be -- but I also wanted to give some hint of the applications that have motivated this work all along. One of these applications is to cybernetic systems, whether we see these systems as agents or cultures, individuals or species, organisms or organizations. A cybernetic system has goals and actions for reaching them. It has a state space X, giving us all of the states that the system can be in, plus it has a goal space G c X, the set of states that the system "likes" to be in, in other words, the distinguished subset of possible states where the system is regarded as living, surviving, or thriving, depending on the type of goal that one has in mind for the system in question. As for actions, there is to begin with the full set !T! of all possible actions, each of which is a transformation of the form T : X -> X, but a given cybernetic system will most likely have but a subset of these actions available to it at any given time. And even if we begin by thinking of actions in very general and very global terms, as arbitrarily complex transformations acting on the whole state space X, we quickly find a need to analyze and approximate them in terms of simple transformations acting locally. The preferred measure of "simplicity" will of course vary from one paradigm of research to another. A generic enough picture at this stage of the game, and one that will remind us of these fundamental features of the cybernetic system even as things get far more complex, is afforded by Figure 23. o---------------------------------------------------------------------o | | | X | | o-------------------o | | / \ | | / \ | | / \ | | / \ | | / \ | | / \ | | / \ | | o o | | | | | | | | | | | | | | | G | | | | | | | | | | | | | | | o o | | \ / | | \ / | | \ T / | | \ o<------------/-------------o | | \ / | | \ / | | \ / | | o-------------------o | | | | | o---------------------------------------------------------------------o Figure 23. Elements of a Cybernetic System Note 24 Now that we've introduced the field picture for thinking about propositions and their analytic series, a very pleasing way of picturing the relationship among a proposition f : X -> B, its enlargement or shift map Ef : EX -> B, and its difference map Df : EX -> B can now be drawn. To illustrate this possibility, let's return to the differential analysis of the conjunctive proposition f<p, q> = pq, giving the development a slightly different twist at the appropriate point. Figure 24-1 shows the proposition pq once again, which we now view as a scalar field, in effect, a potential "plateau" of elevation 1 over the shaded region, with an elevation of 0 everywhere else. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / o \ | | / /%\ \ | | / /%%%\ \ | | / /%%%%%\ \ | | / /%%%%%%%\ \ | | / /%%%%%%%%%\ \ | | o o%%%%%%%%%%%o o | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | P |%%%%%%%%%%%| Q | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | | |%%%%%%%%%%%| | | | o o%%%%%%%%%%%o o | | \ \%%%%%%%%%/ / | | \ \%%%%%%%/ / | | \ \%%%%%/ / | | \ \%%%/ / | | \ \%/ / | | \ o / | | \ / \ / | | o-------------------o o-------------------o | | | | | o---------------------------------------------------------------------o Figure 24-1. Proposition pq : X -> B Given any proposition f : X -> B, the "tacit extension" of f to EX is notated !e!f : EX -> B and defined by the equation !e!f = f, so it's really just the same proposition living in a bigger universe. Tacit extensions formalize the intuitive idea that a new function is related to an old function in such a way that it obeys the same constraints on the old variables, with a "don't care" condition on the new variables. Figure 24-2 illustrates the "tacit extension" of the proposition or scalar field f = pq : X -> B to give the extended proposition or differential field that we notate as !e!f = !e![pq] : EX -> B. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o (dp) (dq) o o | | | | o-->--o | | | | | | \ / | | | | | (dp) dq | \ / | dp (dq) | | | | o<-----------------o----------------->o | | | | | | | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 24-2. Tacit Extension !e![pq] : EX -> B Thus we have a pictorial way of visualizing the following data: !e![pq] = p q . dp dq + p q . dp (dq) + p q . (dp) dq + p q . (dp)(dq) Note 25 Staying with the example pq : X -> B, Figure 25-1 shows the enlargement or shift map E[pq] : EX -> B in the same style of differential field picture that we drew for the tacit extension !e![pq] : EX -> B. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o (dp) (dq) o o | | | | o-->--o | | | | | | \ / | | | | | (dp) dq | \ / | dp (dq) | | | | o----------------->o<-----------------o | | | | | ^ | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | | | | o | | | o---------------------------------------------------------------------o Figure 25-1. Enlargement E[pq] : EX -> B A very important conceptual transition has just occurred here, almost tacitly, as it were. Generally speaking, having a set of mathematical objects of compatible types, in this case the two differential fields !e!f and Ef, both of the type EX -> B, is very useful, because it allows us to consider these fields as integral mathematical objects that can be operated on and combined in the ways that we usually associate with algebras. In this case one notices that the tacit extension !e!f and the enlargement Ef are in a certain sense dual to each other, with !e!f indicating all of the arrows out of the region where f is true, and with Ef indicating all of the arrows into the region where f is true. The only arc that they have in common is the no-change loop (dp)(dq) at pq. If we add the two sets of arcs mod 2, then the common loop drops out, leaving the 6 arrows of D[pq] = !e![pq] + E[pq] that are illustrated in Figure 25-2. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o o o | | | | | | | | | | | | | | | (dp) dq | | dp (dq) | | | | o<---------------->o<---------------->o | | | | | ^ | | | | | | | | | | | | | | | | | | o o | o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ | / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | dp | dq | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 25-2. Difference Map D[pq] : EX -> B The differential features of D[pq] may be collected cell by cell of the underlying universe X% = [p, q] to give the following expansion: D[pq] = p q . ((dp)(dq)) + p (q) . (dp) dq + (p) q . dp (dq) + (p)(q) . dp dq Note 26 If we follow the classical line that singles out linear functions as ideals of simplicity, then we may complete the analytic series of the proposition f = pq : X -> B in the following way. Figure 26-1 shows the differential proposition df = d[pq] : EX -> B that we get by extracting the cell-wise linear approximation to the difference map Df = D[pq] : EX -> B. This is the logical analogue of what would ordinarily be called 'the' differential of pq, but since I've been attaching the adjective "differential" to just about everything in sight, the distinction tends to be lost. For the time being, I'll resort to using the alternative name "tangent map" for df. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / o \ \ | | / / ^ ^ \ \ | | o o / \ o o | | | | / \ | | | | | | / \ | | | | | |/ \| | | | | (dp)/ dq dp \(dq) | | | | /| |\ | | | | / | | \ | | | | / | | \ | | | o / o o \ o | | \ v \ dp dq / v / | | \ o<--------------------->o / | | \ \ / / | | \ \ / / | | \ \ / / | | \ o / | | \ / \ / | | o-------------------o o-------------------o | | | | | o---------------------------------------------------------------------o Figure 26-1. Differential or Tangent d[pq] : EX -> B Just to be clear about what's being indicated here, it's a visual way of specifying the following data: d[pq] = p q . (dp, dq) + p (q) . dq + (p) q . dp + (p)(q) . 0 To understand the extended interpretations, that is, the conjunctions of basic and differential features that are being indicated here, it may help to note the following equivalences: (dp, dq) = dp + dq = dp(dq) + (dp)dq dp = dp dq + dp(dq) dq = dp dq + (dp)dq Capping the series that analyzes the proposition pq in terms of succeeding orders of linear propositions, Figure 26-2 shows the remainder map r[pq] : EX -> B, that happens to be linear in pairs of variables. o---------------------------------------------------------------------o | | | X | | o-------------------o o-------------------o | | / \ / \ | | / P o Q \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | / / \ \ | | o o o o | | | | | | | | | | | | | | | | dp dq | | | | | o<------------------------------->o | | | | | | | | | | | | | | | | | o | | | | o o ^ o o | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \ | / / | | \ \|/ / | | \ dp | dq / | | \ /|\ / | | o-------------------o | o-------------------o | | | | | | | | | | | v | | o | | | o---------------------------------------------------------------------o Figure 26-2. Remainder r[pq] : EX -> B Reading the arrows off the map produces the following data: r[pq] = p q . dp dq + p (q) . dp dq + (p) q . dp dq + (p)(q) . dp dq In short, r[pq] is a constant field, having the value dp dq at each cell. A more detailed presentation of Differential Logic can be found here: DLOG D. http://stderr.org/pipermail/inquiry/2003-May/thread.html#478 DLOG D. http://stderr.org/pipermail/inquiry/2003-June/thread.html#553 DLOG D. http://stderr.org/pipermail/inquiry/2003-June/thread.html#571 Document History Ontology List (Apr–Jul 2002) http://suo.ieee.org/ontology/thrd28.html#04040 http://suo.ieee.org/ontology/msg04040.html http://suo.ieee.org/ontology/msg04041.html http://suo.ieee.org/ontology/msg04045.html http://suo.ieee.org/ontology/msg04046.html http://suo.ieee.org/ontology/msg04047.html http://suo.ieee.org/ontology/msg04048.html http://suo.ieee.org/ontology/msg04052.html http://suo.ieee.org/ontology/msg04054.html http://suo.ieee.org/ontology/msg04055.html http://suo.ieee.org/ontology/msg04067.html http://suo.ieee.org/ontology/msg04068.html http://suo.ieee.org/ontology/msg04069.html http://suo.ieee.org/ontology/msg04070.html http://suo.ieee.org/ontology/msg04072.html http://suo.ieee.org/ontology/msg04073.html http://suo.ieee.org/ontology/msg04074.html http://suo.ieee.org/ontology/msg04077.html http://suo.ieee.org/ontology/msg04079.html http://suo.ieee.org/ontology/msg04080.html http://suo.ieee.org/ontology/msg04268.html http://suo.ieee.org/ontology/msg04269.html http://suo.ieee.org/ontology/msg04272.html http://suo.ieee.org/ontology/msg04273.html http://suo.ieee.org/ontology/msg04290.html Inquiry List (May & Jul 2004) http://stderr.org/pipermail/inquiry/2004-May/thread.html#1400 http://stderr.org/pipermail/inquiry/2004-July/thread.html#1685 http://stderr.org/pipermail/inquiry/2004-May/001400.html http://stderr.org/pipermail/inquiry/2004-May/001401.html http://stderr.org/pipermail/inquiry/2004-May/001402.html http://stderr.org/pipermail/inquiry/2004-May/001403.html http://stderr.org/pipermail/inquiry/2004-May/001404.html http://stderr.org/pipermail/inquiry/2004-May/001405.html http://stderr.org/pipermail/inquiry/2004-May/001406.html http://stderr.org/pipermail/inquiry/2004-May/001407.html http://stderr.org/pipermail/inquiry/2004-May/001408.html http://stderr.org/pipermail/inquiry/2004-May/001410.html http://stderr.org/pipermail/inquiry/2004-May/001411.html http://stderr.org/pipermail/inquiry/2004-May/001412.html http://stderr.org/pipermail/inquiry/2004-May/001413.html http://stderr.org/pipermail/inquiry/2004-May/001415.html http://stderr.org/pipermail/inquiry/2004-May/001416.html http://stderr.org/pipermail/inquiry/2004-May/001418.html http://stderr.org/pipermail/inquiry/2004-May/001419.html http://stderr.org/pipermail/inquiry/2004-May/001420.html http://stderr.org/pipermail/inquiry/2004-May/001421.html http://stderr.org/pipermail/inquiry/2004-May/001422.html http://stderr.org/pipermail/inquiry/2004-May/001423.html http://stderr.org/pipermail/inquiry/2004-May/001424.html http://stderr.org/pipermail/inquiry/2004-July/001685.html http://stderr.org/pipermail/inquiry/2004-July/001686.html http://stderr.org/pipermail/inquiry/2004-July/001687.html http://stderr.org/pipermail/inquiry/2004-July/001688.html NKS Forum (May & Jul 2004) http://forum.wolframscience.com/archive/topic/420-1.html http://forum.wolframscience.com/printthread.php?threadid=420 http://forum.wolframscience.com/showthread.php?threadid=420 http://forum.wolframscience.com/showthread.php?postid=1282#post1282 http://forum.wolframscience.com/showthread.php?postid=1285#post1285 http://forum.wolframscience.com/showthread.php?postid=1289#post1289 http://forum.wolframscience.com/showthread.php?postid=1292#post1292 http://forum.wolframscience.com/showthread.php?postid=1293#post1293 http://forum.wolframscience.com/showthread.php?postid=1294#post1294 http://forum.wolframscience.com/showthread.php?postid=1296#post1296 http://forum.wolframscience.com/showthread.php?postid=1299#post1299 http://forum.wolframscience.com/showthread.php?postid=1301#post1301 http://forum.wolframscience.com/showthread.php?postid=1304#post1304 http://forum.wolframscience.com/showthread.php?postid=1307#post1307 http://forum.wolframscience.com/showthread.php?postid=1309#post1309 http://forum.wolframscience.com/showthread.php?postid=1311#post1311 http://forum.wolframscience.com/showthread.php?postid=1314#post1314 http://forum.wolframscience.com/showthread.php?postid=1315#post1315 http://forum.wolframscience.com/showthread.php?postid=1318#post1318 http://forum.wolframscience.com/showthread.php?postid=1321#post1321 http://forum.wolframscience.com/showthread.php?postid=1323#post1323 http://forum.wolframscience.com/showthread.php?postid=1326#post1326 http://forum.wolframscience.com/showthread.php?postid=1327#post1327 http://forum.wolframscience.com/showthread.php?postid=1330#post1330 http://forum.wolframscience.com/showthread.php?postid=1331#post1331 http://forum.wolframscience.com/showthread.php?postid=1598#post1598 http://forum.wolframscience.com/showthread.php?postid=1601#post1601 http://forum.wolframscience.com/showthread.php?postid=1602#post1602 http://forum.wolframscience.com/showthread.php?postid=1603#post1603